Page 42 - Fiber Optic Communications Fund

Electromagnetics and Optics 23

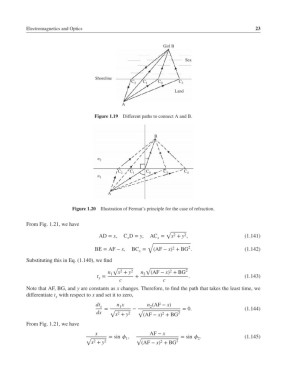
Girl B

Sea

Shoreline
C 2 C 1 C 0 C 3
Land

A
Figure 1.19 Different paths to connect A and B.

B

n 2

C 2 C 1 C 0 C 3 C 4
n 1

A

Figure 1.20 Illustration of Fermat’s principle for the case of refraction.

From Fig. 1.21, we have
√
2
2
AD = x, C D = y, AC = x + y , (1.141)
x
x
√
2
2
BE = AF − x, BC = (AF − x) + BG . (1.142)
x
Substituting this in Eq. (1.140), we find
√ √ 2
2
n 1 x + y 2 n 2 (AF − x) + BG
2
t = + . (1.143)
x
c c
Note that AF, BG, and y are constants as x changes. Therefore, to find the path that takes the least time, we
differentiate t with respect to x and set it to zero,
x
dt x n x n (AF − x)
2
1
= √ − √ = 0. (1.144)
dx x + y 2 (AF − x) + BG 2
2
2
From Fig. 1.21, we have
x AF − x
1
2
√ = sin , √ = sin . (1.145)
2
2
x + y 2 (AF − x) + BG 2