Page 82 - CFA - Day 1 & 2 Course Notes

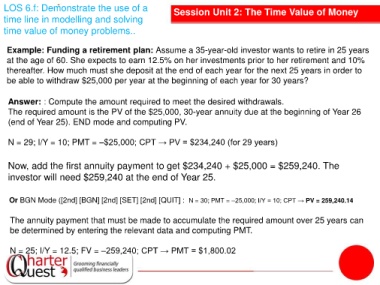
LOS 6.f: Demonstrate the use of a Session Unit 2: The Time Value of Money
time line in modelling and solving

time value of money problems..

Example: Funding a retirement plan: Assume a 35-year-old investor wants to retire in 25 years
at the age of 60. She expects to earn 12.5% on her investments prior to her retirement and 10%

thereafter. How much must she deposit at the end of each year for the next 25 years in order to
be able to withdraw $25,000 per year at the beginning of each year for 30 years?

Answer: : Compute the amount required to meet the desired withdrawals.
The required amount is the PV of the $25,000, 30-year annuity due at the beginning of Year 26

(end of Year 25). END mode and computing PV.

N = 29; I/Y = 10; PMT = –$25,000; CPT → PV = $234,240 (for 29 years)

Now, add the first annuity payment to get $234,240 + $25,000 = $259,240. The

investor will need $259,240 at the end of Year 25.

Or BGN Mode ([2nd] [BGN] [2nd] [SET] [2nd] [QUIT] : N = 30; PMT = –25,000; I/Y = 10; CPT → PV = 259,240.14

The annuity payment that must be made to accumulate the required amount over 25 years can
be determined by entering the relevant data and computing PMT.

N = 25; I/Y = 12.5; FV = –259,240; CPT → PMT = $1,800.02