Page 40 - Mathematics of Business and Finance

20 Chapter 1 | Review of Basic Arithmetic

For example, in finding the GCF of 12 and 18:

■ The factors of 12 are: 1, 2, 3, 4, 6, and 12.
■ The factors of 18 are: 1, 2, 3, 6, 9, and 18.

The common factors are 2, 3, and 6.

Therefore, the GCF of 12 and 18 is 6.
Note: 1 is a factor that is common to all numbers, and therefore we do not bother to include it in the list of
common factors. If there are no common factors other than 1, then 1 is the greatest common factor.

For example, 1 is the only common factor of 3, 5, 7, and 9, and therefore the GCF = 1.
Method 2

First express each number as a product of prime factors. Then identify the set of prime factors that is
common to all the numbers (including repetitions). The product of these prime factors is the GCF.

For example, in finding the GCF of 12 and 18:
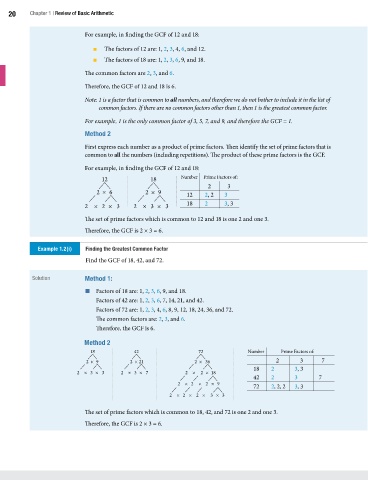
12 18 Number Prime Factors of:
2 3
2 × 6 2 × 9 12 2, 2 3

2 × 2 × 3 2 × 3 × 3 18 2 3, 3
The set of prime factors which is common to 12 and 18 is one 2 and one 3.

Therefore, the GCF is 2 × 3 = 6.

Example 1.2(i) Finding the Greatest Common Factor
Find the GCF of 18, 42, and 72.

Solution Method 1:

■ Factors of 18 are: 1, 2, 3, 6, 9, and 18.
Factors of 42 are: 1, 2, 3, 6, 7, 14, 21, and 42.
Factors of 72 are: 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, and 72.
The common factors are: 2, 3, and 6.
Therefore, the GCF is 6.

Method 2
18 42 72 Number Prime Factors of:
2 × 9 2 × 21 2 × 36 2 3 7
18 2 3,3
2 × 3 × 3 2 × 3 × 7 2 × 2 × 18
42 2 3 7
2 × 2 × 2 × 9 72 2, 2, 2 3,3
2 × 2 × 2× 3 × 3

The set of prime factors which is common to 18, 42, and 72 is one 2 and one 3.
Therefore, the GCF is 2 × 3 = 6.