Page 41 - Mathematics of Business and Finance

Chapter 1 | Review of Basic Arithmetic 21

Example 1.2(j) Finding the Greatest Common Factor to Solve a Word Problem
Three pieces of timber with lengths 24 cm, 36 cm, and 48 cm are to be cut into smaller pieces of
equal length without remainders.
(i) What is the greatest possible length of each piece?
(ii) How many pieces of such equal lengths are possible?

Solution
Method 1
■ Factors of 24 are: 1, 2, 3, 4, 6, 8, 12, and 24.
Factors of 36 are: 1, 2, 3, 4, 6, 9, 12, 18, and 36.
Factors of 48 are: 1, 2, 3, 4, 6, 8, 12, 16, 24, and 48.
The common factors are: 2, 3, 4, 6, and 12.
Therefore, the GCF is 12.

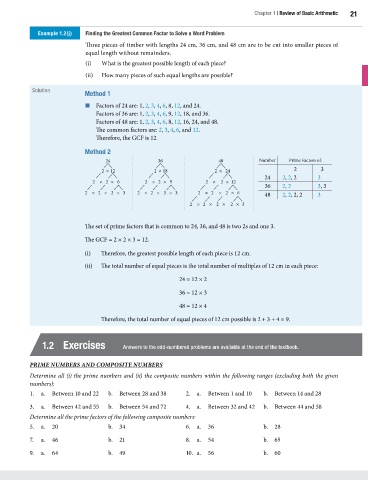
Method 2
24 36 48 Number Prime Factors of:
2 × 12 2 × 18 2 × 24 2 3
24 2, 2, 2 3
2 × 2 × 6 2 × 2 × 9 2 × 2 × 12
36 2, 2 3, 3
2 × 2 × 2 × 3 2 × 2 × 3 × 3 2 × 2 × 2 × 6 48 2, 2, 2, 2 3
2 × 2 × 2× 2 × 3

The set of prime factors that is common to 24, 36, and 48 is two 2s and one 3.

The GCF = 2 × 2 × 3 = 12.
(i) Therefore, the greatest possible length of each piece is 12 cm.

(ii) The total number of equal pieces is the total number of multiples of 12 cm in each piece:
24 = 12 × 2

36 = 12 × 3

48 = 12 × 4

Therefore, the total number of equal pieces of 12 cm possible is 2 + 3 + 4 = 9.

1.2 Exercises Answers to the odd-numbered problems are available at the end of the textbook.

PRIME NUMBERS AND COMPOSITE NUMBERS
Determine all (i) the prime numbers and (ii) the composite numbers within the following ranges (excluding both the given
numbers):
1. a. Between 10 and 22 b. Between 28 and 38 2. a. Between 1 and 10 b. Between 14 and 28

3. a. Between 42 and 55 b. Between 54 and 72 4. a. Between 32 and 42 b. Between 44 and 58
Determine all the prime factors of the following composite numbers:
5. a. 20 b. 34 6. a. 36 b. 28

7. a. 46 b. 21 8. a. 54 b. 65
9. a. 64 b. 49 10. a. 56 b. 60