Page 3 - Vibrations 3

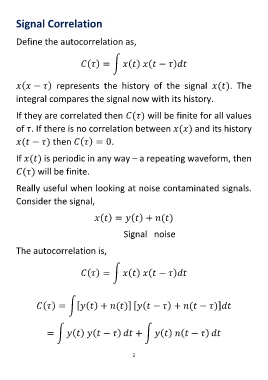
Signal Correlation

Define the autocorrelation as,

() = ∫ () ( − )

( − ) represents the history of the signal (). The

integral compares the signal now with its history.

If they are correlated then () will be finite for all values

of . If there is no correlation between () and its history

( − ) then () = 0.

If () is periodic in any way – a repeating waveform, then

() will be finite.

Really useful when looking at noise contaminated signals.

Consider the signal,

() = () + ()

Signal noise

The autocorrelation is,

() = ∫ () ( − )

() = ∫[() + ()] [( − ) + ( − )]

= ∫ () ( − ) + ∫ () ( − )

2