Page 138 - Maxwell House

118 Chapter 3

3.1.3 Voltage, Current and Power Loss

Apparently, the first integral on the right-hand side of (3.9) related to material conductivity
2
describes the Ohmic loss () = ∫ due to the power converted into heat or any

other than EM kind of energy. Considering the dot-product ∘ > 0 in small conductive
cylinder as shown in Figure 3.1.1a and using the fact that = we can define the power
loss in conventional terms of electrical current , voltage and resistivity R

() = ∫ ∘ = ∫ ( ∘ ) ( ∘ ) =
��������� �������

� (3.11)
2
2
= = ⁄

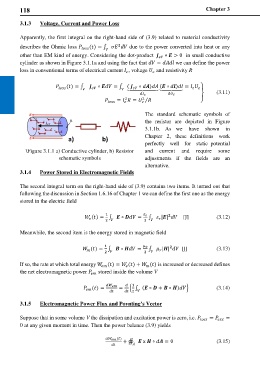
The standard schematic symbols of
the resistor are depicted in Figure
3.1.1b. As we have shown in
a) b) Chapter 2, these definitions work
perfectly well for static potential
\Figure 3.1.1 a) Conductive cylinder, b) Resistor and current and require some
schematic symbols adjustments if the fields are an
alternative.
3.1.4 Power Stored in Electromagnetic Fields

The second integral term on the right-hand side of (3.9) contains two items. It turned out that
following the discussion in Section 1.6.16 of Chapter 1 we can define the first one as the energy
stored in the electric field

1
() = ∫ ∘ = ∫ || [J] (3.12)

2 2
Meanwhile, the second item is the energy stored in magnetic field

1 0
() = ∫ ∘ = ∫ || [J] (3.13)

2 2
If so, the rate at which total energy () = () + () is increased or decreased defines

the net electromagnetic power stored inside the volume V

1
() = = � ∫ ( ∘ + ∘ )� (3.14)

2
3.1.5 Electromagnetic Power Flux and Poynting’s Vector
Suppose that in some volume V the dissipation and excitation power is zero, i.e. = =
0 at any given moment in time. Then the power balance (3.9) yields

() (3.15)
+ ∯ x ∘ = 0