Page 143 - Maxwell House

POYNTING's THEOREM 123

Although there are only static fields, the Poynting vector ≠ 0 (blue vector). In this case, the
nonzero Poynting vector just indicates that such field
assembly causes the static pressure on the bodies placed
nearby and thereby capable to move them. This
phenomena is the core of one of the most important
th
invention of 20 century, cavity magnetron, giving the
allied air forces decisive air supremacy and thereby helps
them to “win” the Second World War. The cavity
magnetrons are the “high-power hearts” of not only old
but many modern microwave radars. Today they are in
mass production being an integral part of peaceful Figure 3.1.7 Static electric and
microwave ovens. magnetic field added together

3.1.8 Angular Momentum of EM Fields. Polarization. Twisted EM Waves

The quantum mechanics teaches us that the probability to
find a photon at a particular location is proportional to the
intensity of the EM wave at that spot, i.e. equivalent to .
2
Besides, there must be at last two different kinds of photons,
ones are in the based state corresponding to a wave with
vertical orientation of E-vector while another ones are in the
base state corresponding to a horizontal orientation of the
same vector. Again, the probability defines this orientation.
According to Figure 3.1.4, as long as all such photons travel
over the radial direction, = 0 and the angular moment is
⊥
zero too. Therefore, EM wave formed by an innumerable
number of these photons keeps a vertical or horizontal
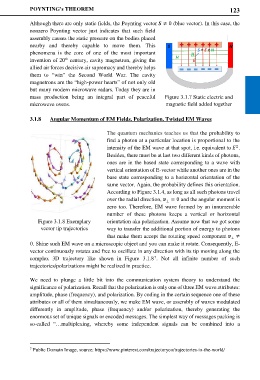
Figure 3.1.8 Exemplary orientation aka polarization. Assume now that we got some
vector tip trajectories way to transfer the additional portion of energy to photons

that make them accept the rotating speed component ≠
⊥
0. Shine such EM wave on a microscopic object and you can make it rotate. Consequently, E-
vector continuously rotates and free to oscillate in any direction with its tip moving along the
complex 3D trajectory like shown in Figure 3.1.8 . Not all infinite number of such
7
trajectories/polarizations might be realized in practice.

We need to plunge a little bit into the communication system theory to understand the
significance of polarization. Recall that the polarization is only one of three EM wave attributes:
amplitude, phase (frequency), and polarization. By coding in the certain sequence one of these
attributes or all of them simultaneously, we make EM wave, or assembly of waves modulated
differently in amplitude, phase (frequency) and/or polarization, thereby generating the
enormous set of unique signals or encoded messages. The simplest way of messages packing is
so-called “…multiplexing, whereby some independent signals can be combined into a

7 Public Domain Image, source: https://www.pinterest.com/trajectoryco/trajectories-in-the-world/