Page 147 - Maxwell House

POYNTING's THEOREM 127

a) b) c)

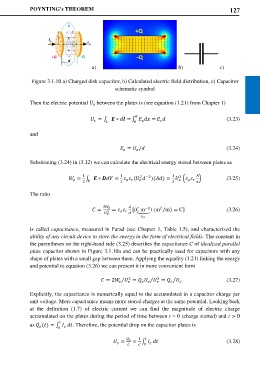
Figure 3.1.10 a) Charged disk capacitor, b) Calculated electric field distribution, c) Capacitor
schematic symbol

Then the electric potential between the plates is (see equation (1.21) from Chapter 1)

= ∫ ∘ = ∫ = (3.23)

0
and
= / (3.24)

Substituting (3.24) in (3.12) we can calculate the electrical energy stored between plates as
1 1 2 −2 1 2
= ∫ ∘ = ( )() = � � (3.25)

2 2 2
The ratio

2
−1
2
= = [(C m���) (m /m) = C] (3.26)
2

is called capacitance, measured in Farad (see Chapter 1, Table 1.5), and characterized the
ability of any circuit device to store the energy in the form of electrical fields. The constant in
the parentheses on the right-hand side (3.25) describes the capacitance C of idealized parallel
plate capacitor shown in Figure 3.1.10a and can be practically used for capacitors with any
shape of plates with a small gap between them. Applying the equality (1.21) linking the energy
and potential to equation (3.26) we can present it in more convenient form

= 2 = = (3.27)
2
2
⁄
⁄
⁄

Explicitly, the capacitance is numerically equal to the accumulated in a capacitor charge per
unit voltage. More capacitance means more stored charges at the same potential. Looking back
at the definition (1.7) of electric current we can find the magnitude of electric charge
accumulated on the plates during the period of time between t = 0 (charge started) and t > 0

as () = ∫ . Therefore, the potential drop on the capacitor plates is

0
1
= = ∫ (3.28)

0