Page 148 - Maxwell House

128 Chapter 3

Here then is one more equation of the circuit theory. The schematic symbol of the capacitor is
shown in Figure 3.1.10c. Finally, note that the capacitance in (3.26) should be slightly less than
the real measured one because we omitted the part of electric energy protruded from the plates.
The correct equations are quite complicated and include the special functions of high
mathematics. The simplest way of evaluation is to replace in (3.26) the physical plate area A
with slightly bigger effective value > . Such substitute is equivalent to the up adjustment

of energy concentrated between plates. The quite accurate quasi-empirical equation for
9 = 1 + ( )[ln(16 ) − 1]. As before, ≪ .
capacitance can be written if ⁄ ⁄ ⁄

3.1.12 Concept of Inductance

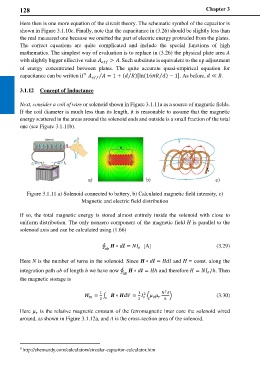
Next, consider a coil of wire or solenoid shown in Figure 3.1.11a as a source of magnetic fields.
If the coil diameter is much less than its length, it is reasonable to assume that the magnetic
energy scattered in the areas around the solenoid ends and outside is a small fraction of the total
one (see Figure 3.1.11b).

a) b) c)

Figure 3.1.11 a) Solenoid connected to battery, b) Calculated magnetic field intensity, c)
Magnetic and electric field distribution

If so, the total magnetic energy is stored almost entirely inside the solenoid with close to
uniform distribution. The only nonzero component of the magnetic field H is parallel to the
solenoid axis and can be calculated using (1.66)

∮ ∘ = [A] (3.29)

Here N is the number of turns in the solenoid. Since ∘ = and H = const. along the
integration path ab of length h we have now ∮ ∘ = ℎ and therefore = /ℎ. Then

the magnetic storage is

2
1 1 2
= ∫ ∘ = � � (3.30)

2 2 0 ℎ
Here is the relative magnetic constant of the ferromagnetic inter core the solenoid wired

around, as shown in Figure 3.1.12a, and A is the cross-section area of the solenoid.

9 http://chemandy.com/calculators/circular-capacitor-calculator.htm