Page 151 - Maxwell House

POYNTING's THEOREM 131

electromagnetic field. It is worth to note the certain symmetry in the structure of equations
(3.41) and (3.38).

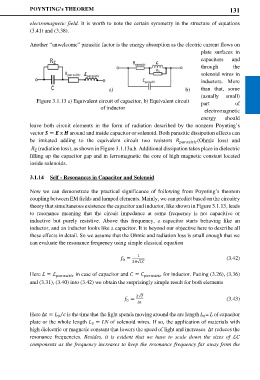
Another “unwelcome” parasitic factor is the energy absorption as the electric current flows on
plate surfaces in
capacitors and
through the
solenoid wires in
inductors. More
a) b) than that, some
(usually small)
Figure 3.1.13 a) Equivalent circuit of capacitor, b) Equivalent circuit part of
of inductor
electromagnetic
energy should
leave both circuit elements in the form of radiation described by the nonzero Poynting’s
vector = x around and inside capacitor or solenoid. Both parasitic dissipation effects can
be imitated adding to the equivalent circuit two resistors (Ohmic loss) and
(radiation loss), as shown in Figure 3.1.13a,b. Additional dissipation takes place in dielectric
Σ
filling up the capacitor gap and in ferromagnetic the core of high magnetic constant located
inside solenoids.

3.1.14 Self - Resonances in Capacitor and Solenoid

Now we can demonstrate the practical significance of following from Poynting’s theorem
coupling between EM fields and lumped elements. Mainly, we can predict based on the circuitry
theory that simultaneous existence the capacitor and inductor, like shown in Figure 3.1.13, leads
to resonance meaning that the circuit impedance at some frequency is not capacitive or
inductive but purely resistive. Above this frequency, a capacitor starts behaving like an
inductor, and an inductor looks like a capacitor. It is beyond our objective here to describe all
these effects in detail. So we assume that the Ohmic and radiation loss is small enough that we
can evaluate the resonance frequency using simple classical equation

1
= (3.42)
0
2√ℒ
Here ℒ = ℒ in case of capacitor and = for inductor. Putting (3.26), (3.36)
and (3.31), (3.40) into (3.42) we obtain the surprisingly simple result for both elements

2√2
= (3.43)
0
Δ
Here Δ = ⁄ is the time that the light spends moving around the arc length = L of capacitor
0
0
plate or the whole length = LN of solenoid wires. If so, the application of materials with
0
high dielectric or magnetic constant that lowers the speed of light and increases Δ reduces the
resonance frequencies. Besides, it is evident that we have to scale down the sizes of ℒC
components as the frequency increases to keep the resonance frequency far away from the