Page 142 - Maxwell House

122 Chapter 3

6
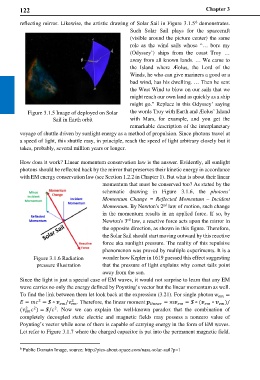
reflecting mirror. Likewise, the artistic drawing of Solar Sail in Figure 3.1.5 demonstrates.
Such Solar Sail plays for the spacecraft
(visible around the picture center) the same
role as the wind sails whose “… bore my
(Odyssey’) ships from the coast Troy …
away from all known lands. … We came to
the Island where Æolus, the Lord of the
Winds, he who can give mariners a good or a
bad wind, has his dwelling. … Then he sent
the West Wind to blow on our sails that we
might reach our own land as quickly as a ship
might go.” Replace in this Odyssey’ saying
Figure 3.1.5 Image of deployed on Solar the words Troy with Earth and Æolus’ Island
Sail in Earth orbit with Mars, for example, and you get the
remarkable description of the interplanetary
voyage of shuttle driven by sunlight energy as a method of propulsion. Since photons travel at
a speed of light, this shuttle may, in principle, reach the speed of light arbitrary closely but it
takes, probably, several million years or longer.

How does it work? Linear momentum conservation law is the answer. Evidently, all sunlight
photons should be reflected back by the mirror that preserves their kinetic energy in accordance
with EM energy conservation law (see Section 1.2.2 in Chapter 1). But what is about their linear
momentum that must be conserved too? As stated by the
schematic drawing in Figure 3.1.6, the photons’
Momentum Change = Reflected Momentum – Incident
nd
Momentum. By Newton's 2 law of motion, such change
in the momentum results in an applied force. If so, by
Newton's 3 law, a reactive force acts upon the mirror in
rd
the opposite direction, as shown in this figure. Therefore,
the Solar Sail should start moving outward by this reactive
force aka sunlight pressure. The reality of this repulsive
phenomenon was proved by multiple experiments. It is a
Figure 3.1.6 Radiation wonder how Kepler in 1619 guessed this effect suggesting
pressure illustration that the pressure of light explains why comet tails point
away from the sun.
Since the light is just a special case of EM waves, it would not surprise to learn that any EM
wave carries no only the energy defined by Poynting’s vector but the linear momentum as well.
To find the link between them let look back at the expression (3.21). For single photon =
2
2
= = ∘ / . Therefore, the linear moment = = ∘ ( ∘ )/
2
2
2
( ) = / . Now we can explain the well-known paradox that the combination of
completely decoupled static electric and magnetic fields may possess a nonzero value of
Poynting’s vector while none of them is capable of carrying energy in the form of EM waves.
Let refer to Figure 3.1.7 where the charged capacitor is put into the permanent magnetic field.
6 Public Domain Image, source: http://pics-about-space.com/nasa-solar-sail?p=1