Page 42 - Maxwell House
P. 42
22 Chapter 1
such images are clear for simple fields and charges distributions they are much messier in more
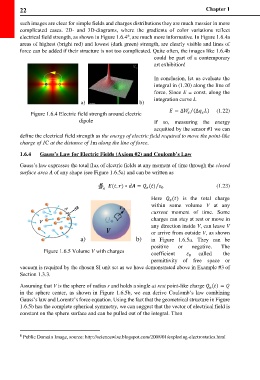
complicated cases. 2D- and 3D-diagrams, where the gradients of color variations reflect
electrical field strength, as shown in Figure 1.6.4 , are much more informative. In Figure 1.6.4a
6
areas of highest (bright red) and lowest (dark green) strength, are clearly visible and lines of
force can be added if their structure is not too complicated. Quite often, the images like 1.6.4b
could be part of a contemporary
art exhibition!
In conclusion, let us evaluate the
integral in (1.20) along the line of
force. Since = const. along the
a) b) integration curve L
Figure 1.6.4 Electric field strength around electric = Δ (Δ )⁄ (1.22)
dipole If so, measuring the energy
acquitted by the sensor #1 we can
define the electrical field strength as the energy of electric field required to move the point-like
charge of 1C at the distance of 1m along the line of force.
1.6.4 Gauss’s Law for Electric Fields (Axiom #2) and Coulomb’s Law
Gauss’s law expresses the total flux of electric fields at any moment of time through the closed
surface area of any shape (see Figure 1.6.5a) and can be written as
∯ (, ) ∘ = () (1.23)
⁄
0
Here () is the total charge
within some volume V at any
current moment of time. Some
charges can stay at rest or move in
any direction inside V, can leave V
or arrive from outside V, as shown
a) b) in Figure 1.6.5a. They can be
positive or negative. The
Figure 1.6.5 Volume V with charges coefficient called the
0
permittivity of free space or
vacuum is required by the chosen SI unit set as we have demonstrated above in Example #3 of
Section 1.3.3.
Assuming that V is the sphere of radius r and holds a single at rest point-like charge () =
in the sphere center, as shown in Figure 1.6.5b, we can derive Coulomb’s law combining
Gauss’s law and Lorentz’s force equation. Using the fact that the geometrical structure in Figure
1.6.5b has the complete spherical symmetry, we can suggest that the vector of electrical field is
constant on the sphere surface and can be pulled out of the integral. Then
6 Public Domain Image, source: http://sciencewise.blogspot.com/2008/01/exploring-electrostatics.html