Page 38 - Maxwell House

18 Chapter 1

derivative = 0 vanishes and the facade and back walls of House becomes decoupled, as

shown in Figure 1.5.1b.
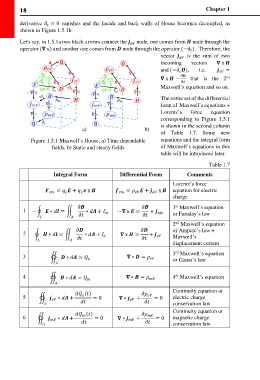
Let's say, in 1.5.1a two black arrows connect the node, one comes from node through the

operator ( x) and another one comes from node through the operator (− ). Therefore, the

vector is the sum of two

0 0 incoming vectors x
and (− ), i.e. =

nd
that is the 2
q q x −
qv - qv Maxwell’s equation and so on.
-
The entire set of the differential
form of Maxwell’s equations +
Lorentz’s force equation
corresponding to Figure 1.5.1
0 0 is shown in the second column
a) b)
of Table 1.7. Some new
Figure 1.5.1 Maxwell’s House, a) Time dependable equations and the integral form
fields, b) Static and steady fields of Maxwell’s equations in this
table will be introduced later.
Table 1.7
Integral Form Differential Form Comments
Lorentz’s force
= + x = + x equation for electric

charge
st
1 Maxwell’s equation
1 − � ∘ = � ∘ + − x = + or Faraday’s law

nd
2 Maxwell’s equation
2 � ∘ = � ∘ + x = + or Ampere’s law +
Maxwell’s

displacement current
3 Maxwell’s equation
rd
3 � ∘ = ∘ = or Gauss’s law

th
4 � ∘ = ∘ = 4 Maxwell’s equation

Continuity equation or
5 � ∘ + () = 0 ∘ + = 0 electric charge

conservation law
Continuity equation or
6 � ∘ + () = 0 ∘ + = 0 magnetic charge

conservation law