Page 34 - Maxwell House

14 Chapter 1

Meanwhile, we know very well that the beams of charged particles can propagate in free space
without any wire support. As an example, we can bring up the solar wind and lightning bolt,
particle beam accelerators and weapon systems, vacuum tubes, and many other natural
phenomena and human-made devices. To include all these phenomena we can associate the
flux of charges with the speed vector pointing in the direction of charge movement and define
the vector as the volume current density

−3
−1
−2
⁄
= ∆ ∆ [(A∙ s ∙ m ) ∙ (m ∙ s ) = A ∙ m ]

(1.3)
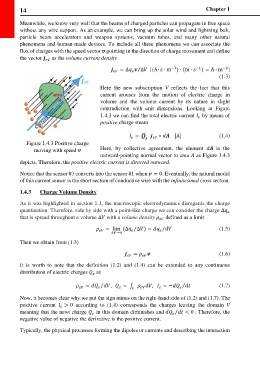
Here the new subscription V reflects the fact that this
current arouses from the motion of electric charge in
volume and the volume current by its nature in slight
contradiction with unit dimensions. Looking at Figure
1.4.3 we can find the total electric current by means of

positive charge steam
= ∯ ∘ [A] (1.4)

Figure 1.4.3 Positive charge
moving with speed Here, by collective agreement, the element is the
outward-pointing normal vector to area as Figure 1.4.3
depicts. Therefore, the positive electric current is directed outward.
Notice that the sensor #3 converts into the sensor #1 when = 0. Eventually, the natural model
of this current sensor is the short section of conductive wire with the infinitesimal cross section.
1.4.3 Charge Volume Density

As it was highlighted in section 1.1, the macroscopic electrodynamics disregards the charge
quantization. Therefore, side by side with a point-like charge we can consider the charge ∆

that is spread throughout a volume ∆ with a volume density defined as a limit

⁄
= lim (∆ ∆) = (1.5)
⁄

∆→0
Then we obtain from (1.3)
= (1.6)

It is worth to note that the definition (1.2) and (1.4) can be extended to any continuous
distribution of electric charges as

⁄
⁄
= , = ∫ , = − (1.7)

Now, it becomes clear why we put the sign minus on the right-hand side of (1.2) and (1.7). The
positive current > 0 according to (1.4) corresponds the charges leaving the domain V

meaning that the newt charge in this domain diminishes and < 0 . Therefore, the
⁄

negative value of negative the derivative is the positive current.
Typically, the physical processes forming the dipoles or currents and describing the interaction