Page 32 - Maxwell House

12 Chapter 1

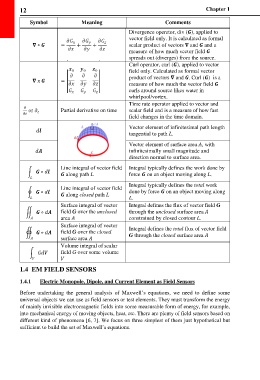
Symbol Meaning Comments
Divergence operator, div (), applied to
vector field only. It is calculated as formal
∘ = + + scalar product of vectors and and a
measure of how much vector field
spreads out (diverges) from the source.
Curl operator, curl (), applied to vector
field only. Calculated as formal vector
� 0 0 0 �
product of vectors and . Curl () is a
x = � �
measure of how much the vector field
curls around source likes water in
whirlpool/vortex.
Time rate operator applied to vector and
or Partial derivative on time scalar field and is a measure of how fast

field changes in the time domain.
Vector element of infinitesimal path length

tangential to path L.
Vector element of surface area , with
infinitesimally small magnitude and
direction normal to surface area.

Line integral of vector field Integral typically defines the work done by
� ∘ along path L force on an object moving along L.

Line integral of vector field Integral typically defines the total work
� ∘ along closed path L done by force on an object moving along
L.
Surface integral of vector Integral defines the flux of vector field G
� ∘ field G over the unclosed through the unclosed surface area
area constrained by closed contour L.

Surface integral of vector Integral defines the total flux of vector field
� ∘ field G over the closed G through the closed surface area
surface area
Volume integral of scalar
� field G over some volume

1.4 EM FIELD SENSORS
1.4.1 Electric Monopole, Dipole, and Current Element as Field Sensors

Before undertaking the general analysis of Maxwell’s equations, we need to define some
universal objects we can use as field sensors or test elements. They must transform the energy
of mainly invisible electromagnetic fields into some measurable form of energy, for example,
into mechanical energy of moving objects, heat, etc. There are plenty of field sensors based on
different kind of phenomena [6, 7]. We focus on three simplest of them just hypothetical but
sufficient to build the set of Maxwell’s equations.