Page 64 - RTH3BA Preview
P. 64
R���� T����� �������� - B������� �� A������� 3 �� E������.
Well, tanϴ=O/A, pops into mind.
Or
Phase angle =tanϴ = X L/R so tanϴ = 10/20 = tan0=0.5
We need to find the arctan (or inverse tangent) of 0.5.
On most calculators, we do this by going (inverse, 2nd, or shift) tan then entering 0.5
and pressing = gives 26.565°
So, the full description of this impedance is then:
Z = 22.36/26.565°Ω
So now we have expressed the impedance consisting of a resistor of 20Ω in series
with an inductive reactance of 10Ω two ways.
For this circuit, the impedance can be expressed (using two numbers) as either.
1. Z=20+j10Ω (this is a complex number in rectangular form)
2. Z=22.36/26.565°Ω (this is the same complex number in polar form)
In rectangular form (1) we have the REAL part + the IMAGINARY part. In polar form
(2) the 22.36 is called the "MAGNITUDE" and the 26.565° is called the ANGLE (or
in our case the phase angle)
Both methods describe the same impedance but using different methods. A modern
impedance meter will give you either or both.
If the reactance was capacitive, we would plot that on the negative imaginary
number line.
How about we do another circuit?
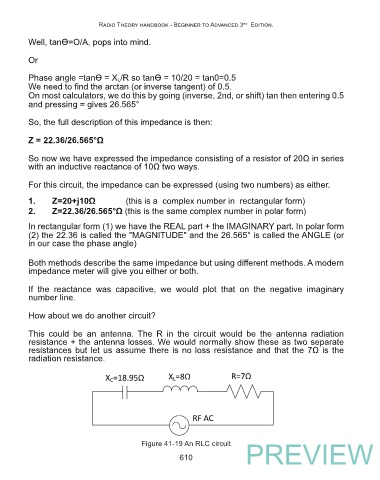
This could be an antenna. The R in the circuit would be the antenna radiation
resistance + the antenna losses. We would normally show these as two separate
resistances but let us assume there is no loss resistance and that the 7Ω is the
radiation resistance.
PREVIEW
Figure 41-19 An RLC circuit
610