Page 68 - RTH3BA Preview

R���� T����� �������� - B������� �� A������� 3 �� E������.

For example, resistances in series, the total resistance is given by:

Rt=R1 + R2 + R3..n
Impedances in series, the total impedance is given by:
Zt=Z1 + Z2 + Z3..n
The total resistance of resistors in parallel is given by the
reciprocal of the sum of the reciprocals:
Rt=1/(1/R1+1/R2+1/R3..n)
Similarly, total impedance of impedances in parallel is given by:
Zt =1/(1/Z1 +1/Z2+1/Z3..n)

Antennas, transmission lines and tuned circuits are just some examples that you can
learn to understand more if you learn how to use complex numbers. Later in this
chapter, we will do some complex number calculations to explain how an antenna
tuner works. I will be using a Casio FX-991ES. Just about all modern scientific
calculators will work in a similar way, but try not to go overboard and purchase a
calculator that is too complex to learn, as you may never use many of the other
advanced functions.

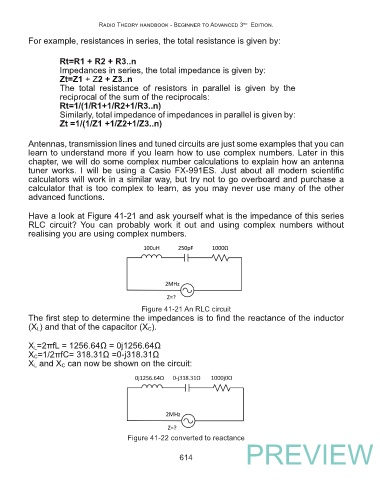
Have a look at Figure 41-21 and ask yourself what is the impedance of this series
RLC circuit? You can probably work it out and using complex numbers without
realising you are using complex numbers.

Figure 41-21 An RLC circuit
The first step to determine the impedances is to find the reactance of the inductor
(X L) and that of the capacitor (X C).

X L=2πfL = 1256.64Ω = 0j1256.64Ω
X C=1/2πfC= 318.31Ω =0-j318.31Ω
X L and X C can now be shown on the circuit:

Figure 41-22 converted to reactance
PREVIEW

614