Page 65 - RTH3BA Preview

41 - ����������� - AC ��������

What is the total impedance of this circuit in ohms?
How much current will flow and how much power is dissipated or radiated?

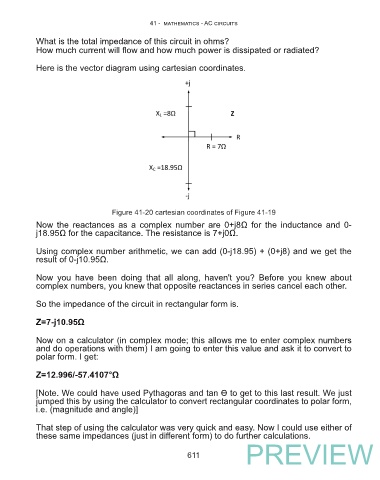
Here is the vector diagram using cartesian coordinates.

Figure 41-20 cartesian coordinates of Figure 41-19
Now the reactances as a complex number are 0+j8Ω for the inductance and 0-
j18.95Ω for the capacitance. The resistance is 7+j0Ω.

Using complex number arithmetic, we can add (0-j18.95) + (0+j8) and we get the
result of 0-j10.95Ω.

Now you have been doing that all along, haven't you? Before you knew about
complex numbers, you knew that opposite reactances in series cancel each other.

So the impedance of the circuit in rectangular form is.

Z=7-j10.95Ω

Now on a calculator (in complex mode; this allows me to enter complex numbers
and do operations with them) I am going to enter this value and ask it to convert to
polar form. I get:

Z=12.996/-57.4107°Ω

[Note. We could have used Pythagoras and tan ϴ to get to this last result. We just
jumped this by using the calculator to convert rectangular coordinates to polar form,
i.e. (magnitude and angle)]

That step of using the calculator was very quick and easy. Now I could use either of
these same impedances (just in different form) to do further calculations.
PREVIEW
611