Page 50 - Nature Of Space And Time

di erent place on future in nity will have a di erent event horizon. Thus event horizons
are a personal matter in de Sitter space.
If one returns to the static form of the de Sitter metric and put = it one gets a
Euclidean metric. There is an apparent singularity on the horizon. However, by de ning
a new radial coordinate and identifying with period 2 , one gets a regular Euclidean
H
metric which is just the four sphere. Because the imaginary time coordinate is periodic, de
Sitter space and all quantum elds in it will behave as if they were at a temperature H .
2
As we shall see, we can observe the consequences of this temperature in the
uctuations in

the microwave background. One can also apply arguments similar to the black hole case
to the action of the Euclidean-de Sitter solution. One nds that it has an intrinsic entropy
of 2 , which is a quarter of the area of the event horizon. Again this entropy arises for
H
a topological reason: the Euler number of the four sphere is two. This means that there
can not be a global time coordinate on Euclidean-de Sitter space. One can interpret this

cosmological entropy as re
ecting an observers lack of knowledge of the universe beyond
his event horizon.

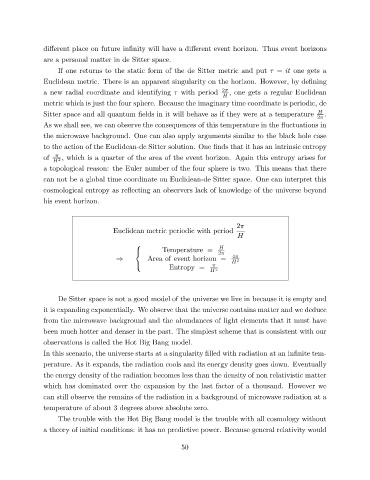
2
Euclidean metric periodic with period
H
 H
Temperature =
 2
⇒ Area of event horizon = 4
H 2
Entropy =

H 2

De Sitter space is not a good model of the universe we live in because it is empty and
it is expanding exponentially. We observe that the universe contains matter and we deduce

from the microwave background and the abundances of light elements that it must have
been much hotter and denser in the past. The simplest scheme that is consistent with our
observations is called the Hot Big Bang model.
In this scenario, the universe starts at a singularity lled with radiation at an in nite tem-

perature. As it expands, the radiation cools and its energy density goes down. Eventually
the energy density of the radiation becomes less than the density of non relativistic matter
which has dominated over the expansion by the last factor of a thousand. However we
can still observe the remains of the radiation in a background of microwave radiation at a

temperature of about 3 degrees above absolute zero.
The trouble with the Hot Big Bang model is the trouble with all cosmology without
a theory of initial conditions: it has no predictive power. Because general relativity would

50