Page 4 - mathematics

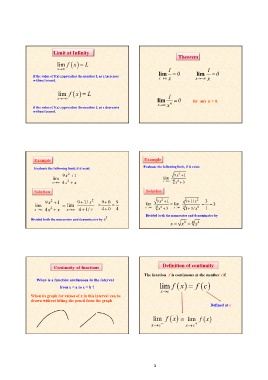
Limit at Infinity Theorem

lim f  x  L lim 1  0 lim 1  0
x x
x x x
if the value of f(x) approaches the number L as x increases
without bound. lim 1 0 for any n > 0.
xn
lim f  x  L x

x

if the value of f(x) approaches the number L as x decreases
without bound.

Example Example

Evaluate the following limit, if it exist: Evaluate the following limit, if it exist:

lim 9x2 1 lim 9 x2 1
4x2  x x 4 x4  3
x

Solution Solution

lim 9 x2 1  lim 91/ x2  9 0  9 lim 9 x2  1  lim 91/ x2  3 3
4 x2  x 41/ x 4 0 4 4 x4  3 x 4 1 3/ x4 1
x x x

Divided both the numerator and denominator by x2 Divided both the numerator and denominator by

x  x2  4 x4

Continuity of functions Definition of continuity

When is a function continuous in the interval The function f is continuous at the number c if
from x = a to x = b ?
lim f  x  f c
When its graph for values of x in this interval can be
drawn without lifting the pencil from the graph xc

Defined at c

lim f  x  lim f  x
xc xc

3