Page 8 - mathematics

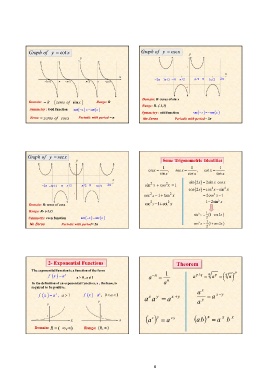
Graph of y  cot x Graph of y  csc x y

y

x 1 x
 / 2  3 / 2 2
3 / 2   / 2  / 2  3 / 2 2 3 / 2   / 2

1

Domain:  R  zeros of sin x  Range: R Domain: R- zeros of sin x

Symmetry : Odd function cot  x   cot x  Range: R- [-1,1]

Symmetry : odd function csc x   csc x 

Zeros:  zeros of cos x Periodic with period = π No Zeros Periodic with period= 2π

Graph of y  sec x y Some Trigonometric Identities

1 cscx  1 x , sec x  1 x , cot x  1 x
sin cos tan
2 3 / 2   / 2
x sin2 x  cos2x 1 sin 2x  2sin x cos x
1  / 2  3 / 2 2 cos 2x  c os2x  sin2 x
sec2x  1 tan2 x
Domain: R- zeros of cosx csc2x 1cot2 x  2cos2 x 1

 1 2sin2 x

Range: R- (-1,1) sin2 x 1 1 cos 2x 
2
Symmetry: even function sec x   sec x  

No Zeros Periodic with period= 2π cos2 x  1 1 cos 2x 
2

2- Exponential Functions Theorem

The exponential function is a function of the form 1  a p / q  q a p  q a p
an
f x  ax a>0,a≠1 an 

In the definition of an exponential function, a , the base, is ax
required to be positive. ay

f x  ax , a 1 f x   ax , 0  a 1 axay  ax y  a x y

y y

1 1 x  ax y  a x y ab x  a x b x

x Range: (0,)

Domain: R  (, )

8