Page 9 - mathematics

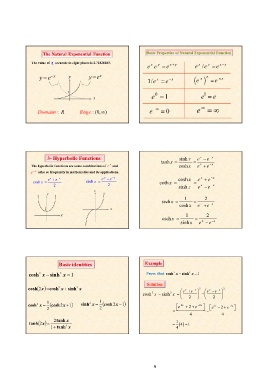
The Natural Exponential Function Basic Properties of Natural Exponential Function

The value of e accurate to eight places is 2.71828183. ex e y exy e x /e y  e x y
1/e x  e x
y  ex y y ex  e x n  e n x

1 e0  1 e1  e
e   0
x e 

Domaim : R Rnge : (0, )

3- Hyperbolic Functions tanh x  sinh x ex  e x
cosh x  ex  e x
The hyperbolic functions are some combinations of e x and
e x arise so frequently in mathematics and its applications.

cosh x  ex  e x sinh x ex e x coth x  cosh x  e x  e x
2  2 sinh x e x e x

y

y

sech x  1 x  ex 2
cosh e x
1 x
x
csch x  1  ex 2
sinh x e x

Basic identities Example
cosh2 x  sinh2 x  1
Prove that cosh2 x  sinh2 x  1

cosh2x cosh2 x  sinh2 x Solution

ex  ex 2  e x e x 2
 2   2 
cosh 2 x  sinh2 x  

1 1 
2 2
cosh 2 x  cosh 2x  1 sinh2 x  cosh 2 x  1 e 2x  2  e 2x   e2x  2  e2x 
4 4


tanh2 x   1 2 tanh x  1  4  1
 tanh 2x 4

9