Page 12 - mathematics

y

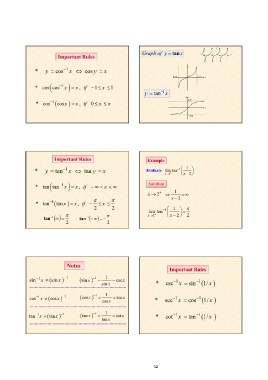
Important Rules Graph of y  tan x 3 /2   / 2 x
 / 2  3 / 2
* y  cos1 x  cos y  x y

 * cos cos1 x  x , if 1  x 1   /2  /2 x

* cos1 cos x   x , if 0  x   y  tan1 x y
 /2
x
 /2

Important Rules Example

* y  tan1 x  tan y  x Evaluate lim tan1  x 1 2 
  
x2

 * tan tan1 x  x , if    x   Solution

x  2  x 1 2 

* tan1tan x   x , if  x 
 2  2 1 
 2
  lim tan 1  x 2  
2 2  
tan 1    tan 1      x2

Notes Important Rules

sin1 x  sin x 1 sin x 1  1  cscx * csc1 x  sin1 1/ x 
sin x * sec1 x  cos1 1/ x 
cos1 x  cos x 1 * cot1 x  tan11/ x 
cos x 1  1 x  sec x
cos

tan1 x   tan x 1 tan x 1  1  cot x
tan x

12