Page 16 - mathematics

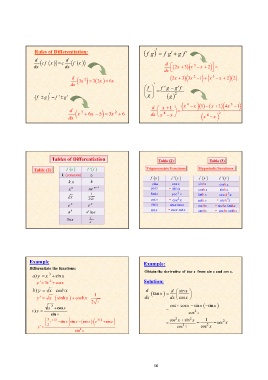
Rules of Differentiation:  f g   f g  g f 

d c f x   c d f x   d
dx dx
dx
 2x  3 x3x 2  


 d 3x 2  32x   6x     2x  3 3x 2 1  x 3  x  2 2

dx  f  f g  gf
 g
f  g   f   g    g 2

   d
 dx
 d  x 1   x4 x 1  x 1 4x 3 1
 x4 x 
dx x4 x 2
x 3  6x  5  3x 2  6

Tables of Differentiation Table (2) Table (3)

Table (1) f x  f x  Trigonometric Functions Hyperbolic Functions

k (constant) 0 f x  f x  f x  f x 

kx k sinx cos x sinhx cosh x
xn nx n 1 cos x sinh x
tan x - sin x cosh x sec h 2x
x 1 tanh x
ex 2x cot x sec2 x - csc h 2x
ax secx coth x - sechx tanhx
ex - csc2 x sechx - cschx cothx
ln x csc x cschx
ax ln a secx tanx

1 - cscx cot x
x

Example Example:

Differentiate the functions: Obtain the derivative of tan x from sin x and cos x.

a) y  x 3  sin x Solution:

y   3x 2  cos x

b ) y  x cosh x 1 d  tan x   d  sin x 
2x dx dx  cos x 
y   x  sinh x   cosh x

x 3  cos x  cos x cos x  sin x sin x 
sin x cos2 x
c)y 

 y  3 1/ 2  cos2 x  sin2 x 1
 2 x  sin x  sin x  cos x  x 3 / 2  cos x  cos2 x  cos2  sec2 x

  sin2 x x

16