Page 17 - mathematics

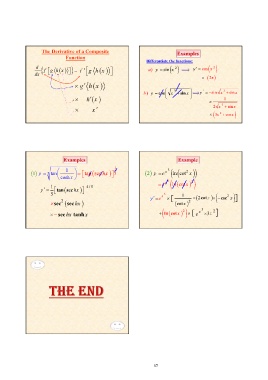
The Derivative of a Composite Examples
Function
Differentiate the functions:

 d  f  g h x    a) y  sin x 2  y   cos x 2

dx
f g h x    2x

 g hx   x3  y '  sin x 3  sin x
  1
b) y  cos  sin x

 hx  2 x 3  sin x

 x   3x 2  cosx

Examples Example

1 y  5 tan  1 x   tan sec hx 1   2 y  e x 3 ln cot2 x
 cosh    e x 3 ln cot x 2
 5

y   1  tan  sec hx 4 / 5
5
 ][y  ex 3 
sec2 sec hx  1 2 2 cot x   csc2 x

cot x

  sec hx tanh x   ][ lncot x 2  e x 3 3x 2

17