Page 19 - mathematics

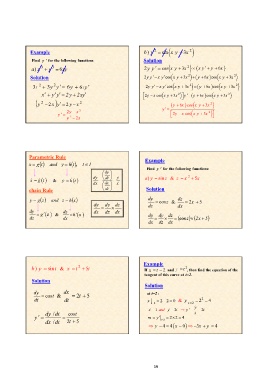
Example  b ) y 2  sin x y  3x 2

Find y ' for the following functions Solution

a) x 3  y 3  6xy  2y y   cos x y  3x 2  x y   y  6x 

Solution    2y y   x y cos x y  3x 2   y  6x cos x y  3x 2

3x 2  3y 2 y   6y  6xy     2y y   x y cos x y  3x 2   y  6x cos x y  3x 2

x2  y2 y  2 y  2xy    2y  x cos
x y  3x 2  y    y  6x cos x y  3x 2

 y 2  2 x y   2 y  x 2
  y  6x  cos x y  3x 2
 y  
y   2y x2 2y  x cos x y  3x 2 
y2  2x 

Parametric Rule Example

x  gt  and y  ht , t  I Find y ' for the following functions:

 dy  a) y  sin z & z  x 2  5x
  
x  g t  & y  h t  dy   dt  y
dx dx x
chain Rule  dt 
Solution

y  gz and z  hx dy dy dz dy  cos z & dz  2x  5
dx dz dx dz dx
 
dy dz
dz  gz  & dx hx  dy  dy  dz  cos z 2x  5 
dx dz dx

b ) y  sint & x  t 2  5t Example
If x  t  2 and y  t 2, then find the equation of the

tangent of this curve at t=2.

Solution Solution

dy  cost & dx  2t 5 at t=2 :
dt dt
x t2  2  2  0 & y t 2  22  4
y
dy / dt cost x  1 and y  2t  y  x  2t
dx / dt 2t  5
y    m  y t2  2  2  4

 y  4  4  x  0  3x  y  4

19