Page 24 - mathematics

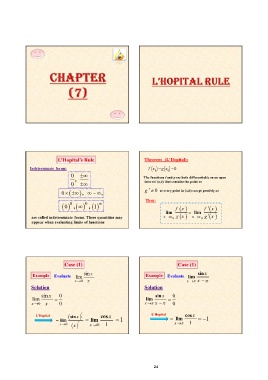
L’Hopital’s Rule Theorem (L'Hopital):

Indeterminate forms: f x0 gx0 0

0 ,  The functions f and g are both differentiable on an open
0  interval (a,b) that contains the point xo

0   ,   , g   0 at every point in (a,b) except possibly xo

00 ,0 , 1 Then:

are called indeterminate forms. These quantities may lim f x   lim f  x 
appear when evaluating limits of functions g x  g  x 
x x 0 x x 0

Case (1) Case (1)

Example Evaluate lim sin x Example Evaluate lim sin x
x x  x  
x 0
Solution
Solution

lim sin x  0 lim sin x  0
x 0 x 0 x  x   0

L’Hopital  sin x  cos x 1 L’Hopital lim cos x  1
1 1
 lim x   lim  x 

x 0 x 0

24