Page 25 - mathematics

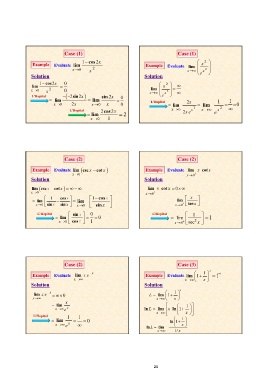
Case (1) Case (1)

Example Evaluate lim 1 cos 2x Example Evaluate lim  x2 
x2  
Solution x 0 Solution x   e x 2 

lim 1  cos 2 x  0  x2  
x2 0  
x 0 lim  e x 2 

L’Hopital lim 2sin2x  sin 2x x  
x
 x 0 2x  lim  0 L’Hopital  lim 2x  lim 1  1 0
0 2x ex 2 ex 2 
x 0 x  x 

L’Hopital  lim 2 cos 2 x 2
1
x 0

Case (2) Case (2)

Example Evaluate lim csc x  cot x  Example Evaluate lim x cot x
x 0
x 0
Solution
Solution

lim csc x  cot x      lim x cot x  0  
x 0
x 0

 lim 1  cos x   lim 1 cos x   lim x 
 sin x sin x   sin x   tan x 
x 0 x 0 x 0

L’Hopital  lim  sin x   0  0 L’Hopital  lim 1  1
 cos x  1 sec2 x 
x 0 x 0

Case (2) Case (3)

Example Evaluate lim x e x Example Evaluate lim  1  1 x  1
 x 
x  x 

Solution Solution

lim x e x    0 L  lim  1  1 x
 x 
x  x x 
ex
 lim ln L  lim  x ln 1 1 
 x 
x  x 

L’Hopital 1  1 0 
ex 
 lim ln  1  1 
x 
x  ln L  lim

x  1/ x

25