Page 13 - mathematics

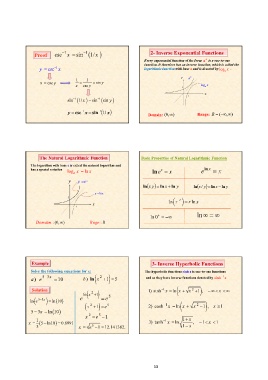
Proof csc1 x  sin1 1/ x  2- Inverse Exponential Functions

y  csc1 x 1  1 y  sin y Every exponential function of the form ax is a one-to-one
x csc
x  csc y function. It therefore has an inverse function, which is called the

logarithmic function with base a and is denoted by loga x .

y ax

1 loga x

x

1

sin1 1/ x   sin1 sin y  Domain: (0,) Range: R  (,)
y  csc1 x  sin11/ x

The Natural Logarithmic Function Basic Properties of Natural Logarithmic Function

The logarithm with base e is called the natural logarithm and ln ex  x eln x  x

has a special notation loge x  ln x lnx y  ln x  ln y lnx / y  ln x  ln y

y y ex

1 y lnx  ln x r  r ln x

1 x

ln 0   ln   

Domaim : (0,) Rnge : R

Example 3- Inverse Hyperbolic Functions

Solve the following equations for x: The hyperbolic functions sinh x is one-to-one functions
and so they have inverse functions denoted by sinh1 x
a ) e 53x  10  b ) ln x 2 1  5
 1) sinh1 x  ln x  x 2 1 ,    x  
Solution  e ln x 2 1  e 5
 x 2 1  e 5
 ln e53x  ln10  2) cosh1 x  ln x  x 2 1 , x 1
x 2 e5 1
5  3x  ln 10
x  e 5  1  12.141382.
x  1 5  ln10  0.8991 3) tanh1 x  ln 1 x , 1 x 1
3 1 x

13