Page 81 - Krugmans Economics for AP Text Book_Neat

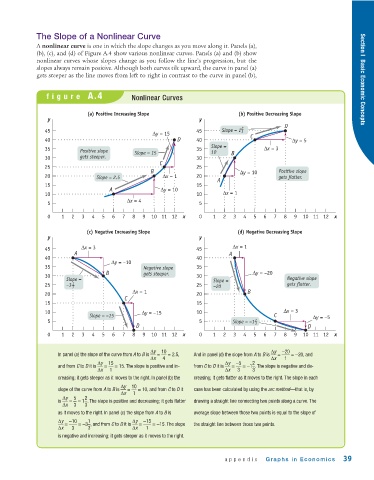
The Slope of a Nonlinear Curve

A nonlinear curve is one in which the slope changes as you move along it. Panels (a),
(b), (c), and (d) of Figure A.4 show various nonlinear curves. Panels (a) and (b) show
nonlinear curves whose slopes change as you follow the line’s progression, but the
slopes always remain positive. Although both curves tilt upward, the curve in panel (a)
gets steeper as the line moves from left to right in contrast to the curve in panel (b), Section I Basic Economic Concepts

figure A.4 Nonlinear Curves

(a) Positive Increasing Slope (b) Positive Decreasing Slope
y y
D
45 45 Slope = 1 3 2
Δy = 15
40 D 40 C Δy = 5
35 Positive slope Slope = 15 35 Slope = Δx = 3
10
30 gets steeper. 30 B
C
25 25
B Δy = 10 Positive slope
20 Slope = 2.5 Δx = 1 20 gets flatter.
A
15 15
A Δy = 10
10 10 Δx = 1
5 Δx = 4 5

0 1 2 3 4 5 6 7 8 9 10 11 12 x 0 1 2 3 4 5 6 7 8 9 10 11 12 x

(c) Negative Increasing Slope (d) Negative Decreasing Slope
y y
45 Δx = 3 45 Δx = 1
A A
40 40
Δy = –10
35 Negative slope 35
30 B gets steeper. 30 Δy = –20
Slope = Slope = Negative slope
25 –3 1 3 25 –20 gets flatter.
20 Δx = 1 20 B
C
15 15
10 Δy = –15 10 Δx = 3
Slope = –15 C Δy = –5
5 5 Slope = –1 2
D 3 D
0 1 2 3 4 5 6 7 8 9 10 11 12 x 0 1 2 3 4 5 6 7 8 9 10 11 12 x

Δy 10 Δy −20
In panel (a) the slope of the curve from A to B is = = 2.5, And in panel (d) the slope from A to B is = = −20, and
Δx 4 Δx 1
Δy 15 Δy −5 2
and from C to D it is = = 15. The slope is positive and in- from C to D it is = = −1 . The slope is negative and de-
Δx 1 Δx 3 3
creasing; it gets steeper as it moves to the right. In panel (b) the creasing; it gets flatter as it moves to the right. The slope in each
Δy 10
slope of the curve from A to B is = = 10, and from C to D it case has been calculated by using the arc method—that is, by
Δx 1
Δy 5 2
is = = 1 . The slope is positive and decreasing; it gets flatter drawing a straight line connecting two points along a curve. The
Δx 3 3
as it moves to the right. In panel (c) the slope from A to B is average slope between those two points is equal to the slope of
Δy −10 1 Δy −15
= = −3 , and from C to D it is = = −15. The slope the straight line between those two points.
Δx 3 3 Δx 1
is negative and increasing; it gets steeper as it moves to the right.

appendix Graphs in Economics 39