Page 73 - Linear Models for the Prediction of Animal Breeding Values

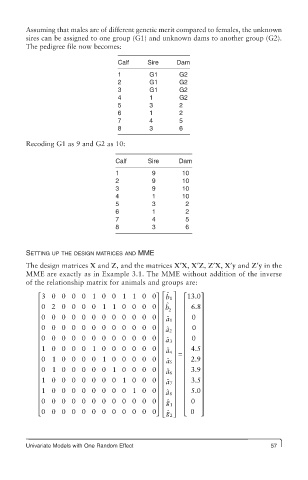
Assuming that males are of different genetic merit compared to females, the unknown
sires can be assigned to one group (G1) and unknown dams to another group (G2).
The pedigree file now becomes:

Calf Sire Dam
1 G1 G2
2 G1 G2
3 G1 G2
4 1 G2
5 3 2
6 1 2
7 4 5
8 3 6
Recoding G1 as 9 and G2 as 10:

Calf Sire Dam
1 9 10
2 9 10
3 9 10
4 1 10
5 3 2
6 1 2
7 4 5
8 3 6

SETTING UP THE DESIGN MATRICES AND MME
The design matrices X and Z, and the matrices X′X, X′Z, Z′X, X′y and Z′y in the
MME are exactly as in Example 3.1. The MME without addition of the inverse
of the relationship matrix for animals and groups are:
⎡ 3 0000 1 0 0 1 1 0 0⎤ ⎡ ˆ ⎤ ⎡13.0⎤
1 b
⎢ ⎥ ⎢ ˆ ⎥ ⎢ ⎢ ⎥
⎢ 0 2 0000 11 0000 ⎥ ⎢ b ⎥ ⎢ 6.8 ⎥
2
⎢ 0 00000000000⎥ ⎢ 1 ˆ a ⎥ ⎢ 0 ⎥
⎢ ⎥ ⎢ ⎥ ⎢ ⎥
⎢ 0 00000000000 ⎢ 2 ˆ a ⎥ ⎢ 0 ⎥
⎥
⎢ 0 000000000000 ⎥ ⎢ ⎥ ⎢ 0 ⎥
⎢ ⎥ ⎢ 3 ˆ a ⎥ ⎢ ⎥
⎢ 10000 1 000000 ⎥ ⎢ ˆ a 4 ⎥ ⎢ 4.5 ⎥
⎥
⎢ 0 1 0000 1 00000 ⎥ ⎢ ⎥ = ⎢ 2.9 ⎥
⎢ ⎥ ⎢ ⎢ 5 ˆ a ⎥ ⎢ ⎥
⎢ 0 1 00000 1 0000⎥ ⎢ 6 ˆ a ⎥ ⎢ 3.9⎥
⎢ ⎥ ⎢ ⎥ ⎢ ⎥
⎢ 10000000 1 000 ⎥ ⎢ 7 ˆ a ⎥ ⎢ 3.5 ⎥
⎢ 1000000000 1 0 0⎥ ⎢ ⎥ ⎢ 5.0⎥
⎢ ⎥ ⎢ 8 ˆ a ⎥ ⎢ ⎥
⎥
⎢ 0 00000000000 ⎢ ˆ g ⎥ ⎢ 0 ⎥
1
⎢ ⎣ 0 00000000000 ⎥ ⎢ ˆ g ⎥ ⎢ ⎣ 0 ⎥ ⎦
⎦ ⎣ 2⎦

Univariate Models with One Random Effect 57