Page 119 - Maxwell House

NEOCLASSICAL THEORY OF INTERACTION 99

to the internet, hearing aids, GPS tracking and smart watches, stress detectors and glucose-
detecting contact lens, etc.
2.9.3 Conductivity of Magnetically Biased Graphene

Graphene is the way to develop reconfigurable devices. According to the plots in Figure 2.9.2
single-atom graphene layer in the microwave range behaves like a moderate conductor.
Nevertheless, it has the
outstanding property of
z y tunability that manifests through
x the electrically regulated number
of conductive electrons or holes.
It can be done by applying to the
layer an external positive or
negative so-called gate voltage.
This classical method of
material conductivity tuning is
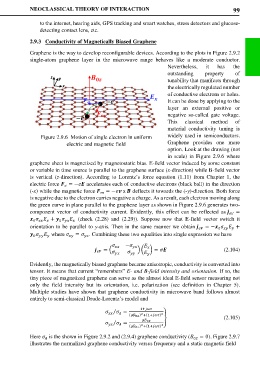
Figure 2.9.6 Motion of single electron in uniform widely used in semiconductors.
electric and magnetic field Graphene provides one more
option. Look at the drawing (not
in scale) in Figure 2.9.6 where
graphene sheet is magnetized by magnetostatic bias. E-field vector induced by some constant
or variable in time source is parallel to the graphene surface (x-direction) while B-field vector
is vertical (z-direction). According to Lorentz’s force equation (1.11) from Chapter 1, the
electric force = − accelerates each of conductive electrons (black ball) in the direction

(-x) while the magnetic force = − x deflects it towards the (-y)-direction. Both force

is negative due to the electron carries negative a charge. As a result, each electron moving along
the green curve in plane parallel to the graphene layer as shown in Figure 2.9.6 generates two-
component vector of conductivity current. Evidently, this effect can be reflected as =

+ (check (2.28) and (2.29)). Suppose now that E-field vector switch it
0
0
orientation to be parallel to y-axis. Then in the same manner we obtain = − +
0
where = . Combining these two equalities into single expression we have

0
−
= � � � � = (2.104)
Evidently, the magnetically biased graphene became anisotropic, conductivity is converted into
tensor. It means that current “remembers” E- and B-field intensity and orientation. If so, the
tiny piece of magnetized graphene can serve as the almost ideal E-field sensor measuring not
only the field intensity but its orientation, i.e. polarization (see definition in Chapter 5).
Multiple studies have shown that graphene conductivity in microwave band follows almost
entirely to semi-classical Drude-Lorentz’s model and

1+
⁄ =

(µ 0 ) 2 +(1+) 2
� (2.105)
µ 0
⁄ =

(µ 0 ) 2 +(1+) 2
Here is the shown in Figure 2.9.2 and (2.9.4) graphene conductivity ( 0 = 0). Figure 2.9.7

illustrates the normalized graphene conductivity versus frequency and a static magnetic field