Page 120 - Maxwell House

100 Chapter 2

assuming that the electron mobility is µ = 50, the average relaxation time = 0.135 ∙
0
10 −12 [s], and 0 ≤ 0 ≤ 1 [T]. The solid red and blue lines correspond to | ⁄ |

0

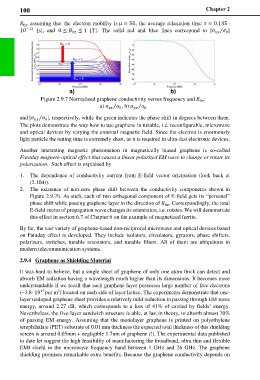
Figure 2.9.7 Normalized graphene conductivity versus frequency and :
0
a) ⁄ , b) ⁄ 0

0

and | ⁄ |, respectively, while the green indicates the phase shift in degrees between them.

0
The plots demonstrate the way how to use graphene in tunable, i.e. reconfigurable, microwave
and optical devices by varying the external magnetic field. Since the electron is enormously
light particle the tuning time is extremely short, as it is required in ultra-fast electronic devices.
Another interesting magnetic phenomenon in magnetically biased graphene is so-called
Faraday magneto-optical effect that causes a linear polarized EM wave to change or rotate its
polarization. Such effect is explained by
1. The dependence of conductivity current from E-field vector orientation (look back at
(2.104)).
2. The existence of non-zero phase shift between the conductivity components shown in
Figure 2.9.7b. As such, each of two orthogonal component of E-field gets its “personal”
phase shift while passing graphene layer in the direction of . Correspondingly, the total
0
E-field vector of propagation wave changes its orientation, i.e. rotates. We will demonstrate
this effect in section 6.7 of Chapter 6 on the example of magnetized ferrite.
By far, the vast variety of graphene-based non-reciprocal microwave and optical devices based
on Faraday effect is developed. They include isolators, circulators, gyrators, phase shifters,
polarizers, switches, tunable resonators, and tunable filters. All of them are ubiquitous in
modern telecommunication systems.

2.9.4 Graphene as Shielding Material
It was hard to believe, but a single sheet of graphene of only one atom thick can detect and
absorb EM radiation having a wavelength much higher than its dimensions. It becomes more
understandable if we recall that such graphene layer possesses large number of free electrons
2
(~3.8∙ 10 per m ) located on each side of layer lattice. The experiments demonstrate that one-
17
layer undoped graphene sheet provides a relatively mild reduction in passing through EM wave
energy, around 2.27 dB, which corresponds to a loss of 41% of carried by fields’ energy.
Nevertheless, the five-layer sandwich structure is able, at last in theory, to absorb almost 70%
of passing EM energy. Assuming that the monolayer graphene is printed on polyethylene
terephthalate (PET) substrate of 0.01 mm thickness the expected total thickness of this shielding
screen is around 0.05mm + negligible 1.7nm of graphene (!). The experimental data published
to date let suggest the high feasibility of manufacturing the broadband, ultra thin and flexible
EMI shield in the microwave frequency band between 1 GHz and 26 GHz. The graphene
shielding promises remarkable extra benefits. Because the graphene conductivity depends on