Page 191 - Maxwell House

SOLUTION OF BASIC EQUATIONS OF ELECTRODYNAMICS 171

Here and is called the magnetic vector and scalar potential, respectively. Evidently,

(4.28) converts into (4.22) and (4.23) when the replacement (4.25) is applied. If the electric and
magnetic current sources are present in Maxwell equations together, we can proceed in three
steps to get the general solution:

1. Solve (4.22) assuming that = 0.

2. Apply (4.23) to the obtained on step1 solution by replacing with the given .

3. Combine these two solutions.
We could be pleased because Maxwell’s equations symmetry saves a lot of computer time and
ours if we exercised numerical analysis.
4.1.6 Electrodynamic Potentials in Space-Frequency Domain

Following in much the same way that led us to equations (4.13), we obtain

2
� 2
∇ (, ) + (, ) = − (, )
0
1 � (4.29)

� 2
2
∇ (, ) + (, ) = − (, )

4.1.7 Green’s Function for Static Fields for Unbounded Space
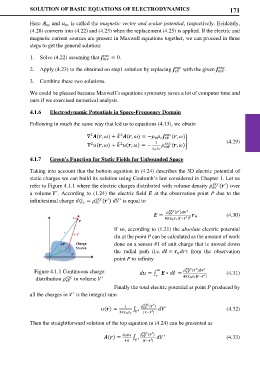
Taking into account that the bottom equation in (4.24) describes the 3D electric potential of
static charges we can build its solution using Coulomb’s law considered in Chapter 1. Let us
′
refer to Figure 4.1.1 where the electric charges distributed with volume density ( ) over

a volume . According to (1.24) the electric field at the observation point P due to the
′
′
′
infinitesimal charge = ( ) is equal to

′ ′
( )
= (4.30)
0
′ 2
4 0 |− |
If so, according to (1.21) the absolute electric potential
at the point P can be calculated as the amount of work
done on a sensor #1 of unit charge that is moved down
the radial path (i.e. = ) from the observation
0
point P to infinity
Figure 4.1.1 Continuous charge = ∫ ∘ = ( ) ′ (4.31)

′
∞
′
in volume 4 0 |− |
′
distribution
Finally the total electric potential at point P produced by
all the charges in is the integral sum
′
′
1 ( ) ′
() = ∫ ′ (4.32)
′
4 0 |− |
Then the straightforward solution of the top equation in (4.24) can be presented as
′
0 ( ) ′
() = ∫ ′ (4.33)
′
4 |− |