Page 454 - Maxwell House

434 Chapter 8

The multilayer design lets produce highly efficient mirrors that are extremely selective and have
very low residual optical absorption loss. In fact, there can be more than 100 layers of material
deposited in the stack. The design principle of such filters is the same as in any filter and based
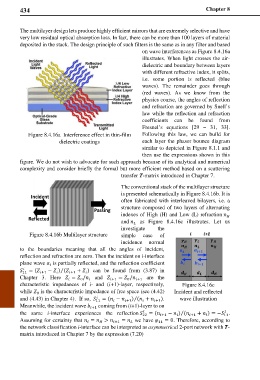
on wave interferences as Figure 8.4.16a
illustrates. When light crosses the air-
dielectric and boundary between layers
with different refractive index, it splits,
i.e. some portion is reflected (blue
waves). The remainder goes through
(red waves). As we know from the
physics course, the angles of reflection
and refraction are governed by Snell’s
law while the reflection and refraction
coefficients can be found from
Fresnel’s equations [29 – 31, 33].
Figure 8.4.16a Interference effect in thin-film Following this law, we can build for
dielectric coatings each layer the phasor bounce diagram
similar to depicted in Figure 8.1.1 and
then use the expressions shown in this
figure. We do not wish to advocate for such approach because of its analytical and numerical
complexity and consider briefly the formal but more efficient method based on a scattering
transfer T-matrix introduced in Chapter 7.
The conventional stack of the multilayer structure
is presented schematically in Figure 8.4.16b. It is
Incident
often fabricated with interleaved bilayers, i.e. a
Passing structure composed of two layers of alternating
Reflected indexes of High (H) and Low (L) refraction

and as Figure 8.4.16c illustrates. Let us

investigate the
Figure 8.4.16b Multilayer structure simple case of
incidence normal
to the boundaries meaning that all the angles of incident,
reflection and refraction are zero. Then the incident on i-interface
plane wave is partially reflected, and the reflection coefficient

⁄
11 = ( +1 − ) ( +1 + ) can be found from (3.87) in

Chapter 3. Here = / and +1 = / +1 are the
0
0

characteristic impedances of i- and (i+1)-layer, respectively, Figure 8.4.16c
while is the characteristic impedance of free space (see (4.42) Incident and reflected
0

⁄
and (4.43) in Chapter 4). If so, 11 = ( − +1 ) ( + +1 ). wave illustration

Meanwhile, the incident wave +1 coming from (i+1)-layer to on

⁄
the same i-interface experiences the reflection 22 = ( +1 − ) ( +1 + ) = − .
11

Assuming for certainty that = > +1 = we have 11 = 0. Therefore, according to

the network classification i-interface can be interpreted as asymmetrical 2-port network with T-
matrix introduced in Chapter 7 by the expression (7.20)