Page 488 - Maxwell House

468 APPENDIX

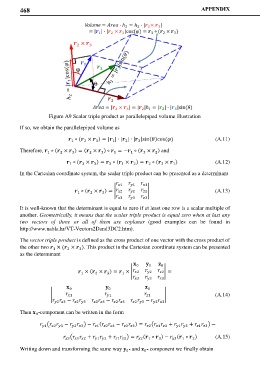
Figure A9 Scalar triple product as parallelepiped volume illustration

If so, we obtain the parallelepiped volume as
∘ ( × ) = | | ∙ | | ∙ | |sin()cos () (A.11)
1 2 3 1 2 3
Therefore, ∘ ( × ) = ( × ) ∘ = − ∘ ( × ) and
1
2
1
2
1
2
3
3
3
∘ ( × ) = ∘ ( × ) = ∘ ( × ) (A.12)
1
3
2
2
1
2
1
3
3
In the Cartesian coordinate system, the scalar triple product can be presented as a determinant
1 1 1
2� (A.13)
∘ ( × ) = � 2 2
3
1
2
3 3 3
It is well-known that the determinant is equal to zero if at least one row is a scalar multiple of
another. Geometrically, it means that the scalar triple product is equal zero when at last any
two vectors of three or all of them are coplanar (good examples can be found in
http://www.nabla.hr/VT-Vectors2Dand3DC2.htm).
The vector triple product is defined as the cross product of one vector with the cross product of
the other two × ( × ). This product in the Cartesian coordinate system can be presented
1
2
3
as the determinant
0 0 0
2� =
× ( × ) = × � 2 2
3
1
1
2
3 3 3
0 0 0
� 1 1 1 � (A.14)
− − −
2 3
2 3
2 3
2 3
2 3
2 3
Then -component can be written in the form
0
� − � − ( − ) = � + + � −
2
1 3
1 3
2 3
2 3
1
1
1 3
2 3
2 3
� + + � = ( ∘ ) − ( ∘ ) (A.15)
3
1
3
1 2
2
3
2
1
1 2
1 2
Writing down and transforming the same way - and - component we finally obtain
0
0