Page 492 - Maxwell House
P. 492
472 APPENDIX
Roughly speaking, the curl measures how much the vector field is rotating around and thus
not going in or out, hence div(curl ) ≡ 0.
curl (grad ) = × ( ∙ ) ≡ 0, since formally ∥ (A.32)
Applying the vector triple product A.16 to × ( × ) the important equity can be proved
2
curl(curl ) = × ( × ) = ∙ ( ∘ ) − ( ∙ ) ∙ = ∙ ( ∘ ) − ∇ ∙ =
grad(div ) − ∇ (A.33)
2
Therefore, in free of electric charges areas where div = 0
curl(curl ) = × ( × ) = −∇ (A.34)
2
Assume that E and H are two vectors. Then div( x ) = ∘ ( x ) can be transferred as
scalar triple product (check the equity (A.12))
div( x ) = ∘ ( x ) = −( ∘ x − ∘ x ) = ∘ curl − ∘ curl (A.35)
Such triple product is the important part of Poynting’s theorem validation. Certainly, it is
possible to prove all of these equities not resorting to help of operator nubla. If our reader is not
convinced and likes to fight, we offer a good exercise to verify them in cylindrical or spherical
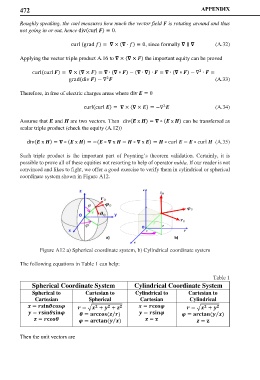
coordinate system shown in Figure A12.
Figure A12 a) Spherical coordinate system, b) Cylindrical coordinate system
The following equations in Table 1 can help:
Table 1
Spherical Coordinate System Cylindrical Coordinate System
Spherical to Cartesian to Cylindrical to Cartesian to
Cartesian Spherical Cartesian Cylindrical
= = � + + = = � +
= = ( ) = = ( )
⁄
⁄
= = ( ) = =
⁄
Then the unit vectors are