Page 489 - Maxwell House

APPENDIX 469

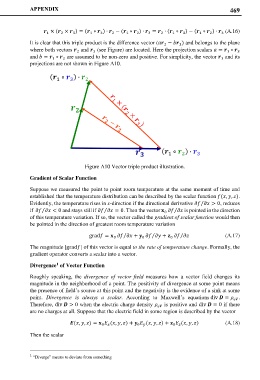
× ( × ) = ( ∘ ) ∙ − ( ∘ ) ∙ = ∙ ( ∘ ) − ( ∘ ) ∙ (A.16)
2
1
1
2
3
2
2
3
1
3
1
1
2
3
3
It is clear that this triple product is the difference vector ( − ) and belongs to the plane
3
2
where both vectors and (see Figure) are located. Here the projection scalars = ∘
2
3
1
3
and = ∘ are assumed to be non-zero and positive. For simplicity, the vector and its
1
2
1
projections are not shown in Figure A10.

Figure A10 Vector triple product illustration.
Gradient of Scalar Function

Suppose we measured the point to point room temperature at the same moment of time and
established that the temperature distribution can be described by the scalar function (, , ).
Evidently, the temperature rises in x-direction if the directional derivative > 0, reduces
⁄
if < 0 and stays still if = 0. Then the vector is pointed in the direction
⁄
⁄
⁄
0
of this temperature variation. If so, the vector called the gradient of scalar function would then
be pointed in the direction of greatest room temperature variation
grad = + + (A.17)
⁄
⁄
⁄
0
0
0
The magnitude |grad| of this vector is equal to the rate of temperature change. Formally, the
gradient operator converts a scalar into a vector.
Divergence of Vector Function
1
Roughly speaking, the divergence of vector field measures how a vector field changes its
magnitude in the neighborhood of a point. The positivity of divergence at some point means
the presence of field’s source at this point and the negativity is the evidence of a sink at some
point. Divergence is always a scalar. According to Maxwell’s equations div = .

Therefore, div > 0 when the electric charge density is positive and div = 0 if there

are no charges at all. Suppose that the electric field in some region is described by the vector
(, , ) = (, , ) + (, , ) + (, , ) (A.18)
0
0
0
Then the scalar
1 “Diverge” means to deviate from something