Page 440 - Mechatronics with Experiments

JWST499-Cetinkunt
JWST499-c07
426 MECHATRONICS Printer: Yet to Come October 9, 2014 8:41 254mm×178mm
By using a smaller weight on side one, we can move a much larger weight on side two. The
circuit acts like a force multiplier. The conservation of energy principle also requires that

Energy In = Energy Out (7.12)
F ⋅ Δx = F ⋅ Δx 2 (7.13)
1
1
2
A
F ⋅ Δx = F ⋅ 2 ⋅ Δx 2 (7.14)
1
1
1
A 1
A ⋅ Δx = A ⋅ Δx 2 (7.15)
1
2
1
A 1
Δx = ⋅ Δx (7.16)
2 1
A
2
which confirms the conservation of fluid volume. As an analogy, this hydraulic circuit acts
like a gear reducer. That is, neglecting the loss of energy due to friction, the energy is con-
served (input energy equal to output energy) and the force is increased while displacement
is reduced.
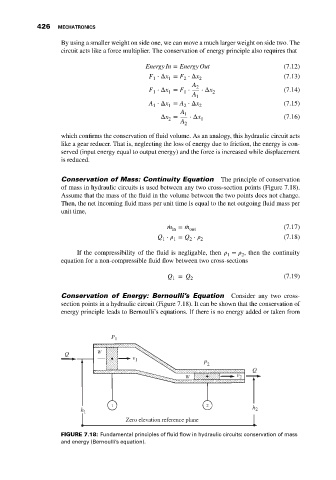
Conservation of Mass: Continuity Equation The principle of conservation
of mass in hydraulic circuits is used between any two cross-section points (Figure 7.18).
Assume that the mass of the fluid in the volume between the two points does not change.
Then, the net incoming fluid mass per unit time is equal to the net outgoing fluid mass per
unit time,
̇ m = ̇ m (7.17)
in out
Q ⋅ = Q ⋅ (7.18)
1 1 2 2
If the compressibility of the fluid is negligable, then = , then the continuity
1
2
equation for a non-compressible fluid flow between two cross-sections
Q = Q (7.19)
1 2
Conservation of Energy: Bernoulli’s Equation Consider any two cross-
section points in a hydraulic circuit (Figure 7.18). It can be shown that the conservation of
energy principle leads to Bernoulli’s equations. If there is no energy added or taken from

P 1

Q W
v 1
P 2
Q
W v 2

1 2 h
h 1 2
Zero elevation reference plane
FIGURE 7.18: Fundamental principles of fluid flow in hydraulic circuits: conservation of mass
and energy (Bernoulli’s equation).