Page 47 - swp0000.dvi

2
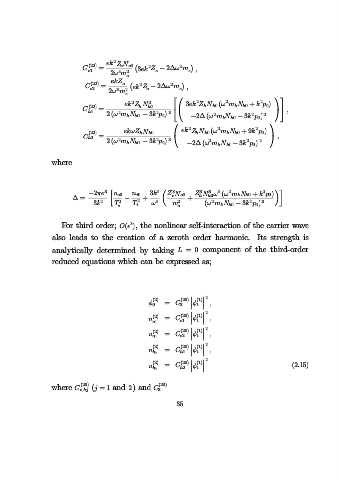
(22)     0 ¡ 2 2 ¢
 1 = 3   − 2∆   
4
2  2

(22)   ¡ 2 2 ¢
 2 =    − 2∆   
3
2  2

⎡⎛ ⎞⎤
2
2
2
2
    2 3    0 (    0 +   0 )
 (22) = 0 ⎣⎝ ⎠⎦ 
1 2 2 3
2(    0 − 3  0 ) −2∆ (    0 − 3  0 ) 2
2
2
⎛ ⎞
2
2
2
    0 (    0 +9  0 )
(22)    0
 = ⎝ ⎠ 
2 2(    0 − 3  0 ) 3 −2∆ (    0 − 3  0 ) 2
2
2
2
2
where
3
3
3
2
4
2
−2 3 ∙  0  0 3 4 µ   0    (    0 +   0 ) ¶¸
∆ = − +  +  0 
2
2
3 2   2   2  4  2  (    0 − 3  0 ) 3
3
For third order; ( ), the nonlinear self-interaction of the carrier wave
also leads to the creation of a zeroth order harmonic. Its strength is
analytically determined by taking  =0 component of the third-order
reduced equations which can be expressed as;
¯ ¯ 2
(2) (20) ¯ (1)¯
 =  ¯ ¯ 
0 3 1
¯ ¯ 2
 (2) =  (20) ¯ (1)¯
¯ ¯ 
 0 1 1
¯ ¯ 2
 (2) =  (20) ¯ (1)¯
¯ ¯ 
 0 2 1
¯ ¯ 2
¯ ¯ 
 (2) =  (20) ¯ (1)¯
 0 1 1
¯ ¯ 2
(2) (20) ¯ (1)¯
 =  ¯ ¯  (2.15)
 0 2 1
where  (20) ( =1 and 2)and  (20)
 3
35