Page 567 - Microeconomics, Fourth Edition

c13marketstructureandcompetition.qxd 7/30/10 1:35 PM Page 541

13.2 OLIGOPOLY WITH HOMOGENEOUS PRODUCTS 541
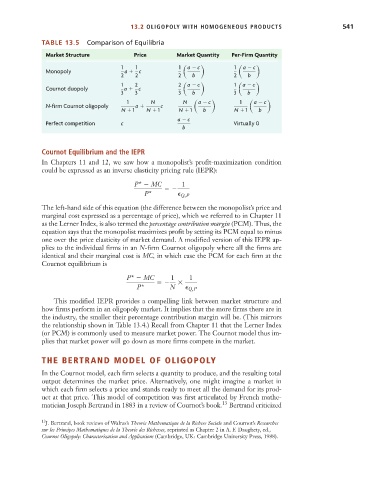
TABLE 13.5 Comparison of Equilibria

Market Structure Price Market Quantity Per-Firm Quantity
1 1 1 a c 1 a c
Monopoly a c
2 2 2 b 2 b
1 2 2 a c 1 a c
Cournot duopoly a c
3 3 3 b 3 b
1 N N a c 1 a c
N -firm Cournot oligopoly a c
N 1 N 1 N 1 b N 1 b
a c
Perfect competition c Virtually 0
b

Cournot Equilibrium and the IEPR
In Chapters 11 and 12, we saw how a monopolist’s profit-maximization condition
could be expressed as an inverse elasticity pricing rule (IEPR):

P* MC 1

P* Q,P
The left-hand side of this equation (the difference between the monopolist’s price and
marginal cost expressed as a percentage of price), which we referred to in Chapter 11
as the Lerner Index, is also termed the percentage contribution margin (PCM). Thus, the
equation says that the monopolist maximizes profit by setting its PCM equal to minus
one over the price elasticity of market demand. A modified version of this IEPR ap-
plies to the individual firms in an N-firm Cournot oligopoly where all the firms are
identical and their marginal cost is MC, in which case the PCM for each firm at the
Cournot equilibrium is

P* MC 1 1

P* N Q,P
This modified IEPR provides a compelling link between market structure and
how firms perform in an oligopoly market. It implies that the more firms there are in
the industry, the smaller their percentage contribution margin will be. (This mirrors
the relationship shown in Table 13.4.) Recall from Chapter 11 that the Lerner Index
(or PCM) is commonly used to measure market power. The Cournot model thus im-
plies that market power will go down as more firms compete in the market.

THE BERTRAND MODEL OF OLIGOPOLY
In the Cournot model, each firm selects a quantity to produce, and the resulting total
output determines the market price. Alternatively, one might imagine a market in
which each firm selects a price and stands ready to meet all the demand for its prod-
uct at that price. This model of competition was first articulated by French mathe-
matician Joseph Bertrand in 1883 in a review of Cournot’s book. 13 Bertrand criticized

13 J. Bertrand, book reviews of Walras’s Theorie Mathematique de la Richese Sociale and Cournot’s Researches
sur les Principes Mathematiques de la Theorie des Richesses, reprinted as Chapter 2 in A. F. Daughety, ed.,
Cournot Oligopoly: Characterization and Applications (Cambridge, UK: Cambridge University Press, 1988).