Page 656 - Microeconomics, Fourth Edition

c15riskandinformation.qxd 8/16/10 11:10 AM Page 630

630 CHAPTER 15 RISK AND INFORMATION

Reservoir is large Oil company's payoff (millions)
Build large (probability = 0.5)
facility $50
(no test)
B Reservoir is small
(probability = 0.5)
$10

Reservoir is large
Build small (probability = 0.5)
facility $30
(no test)
A C Reservoir is small
(probability = 0.5)
$20
Payoff
Build large facility (millions)
Test says reservoir is large
$50
(probability = 0.5)
E Build small facility
Conduct seismic $30
test first
D
Test says reservoir is small Build large facility
(probability = 0.5) $10
F Build small facility
$20
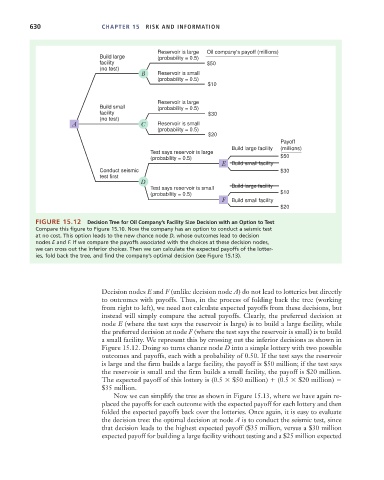
FIGURE 15.12 Decision Tree for Oil Company’s Facility Size Decision with an Option to Test
Compare this figure to Figure 15.10. Now the company has an option to conduct a seismic test
at no cost. This option leads to the new chance node D, whose outcomes lead to decision
nodes E and F. If we compare the payoffs associated with the choices at these decision nodes,
we can cross out the inferior choices. Then we can calculate the expected payoffs of the lotter-
ies, fold back the tree, and find the company’s optimal decision (see Figure 15.13).

Decision nodes E and F (unlike decision node A) do not lead to lotteries but directly
to outcomes with payoffs. Thus, in the process of folding back the tree (working
from right to left), we need not calculate expected payoffs from these decisions, but
instead will simply compare the actual payoffs. Clearly, the preferred decision at
node E (where the test says the reservoir is large) is to build a large facility, while
the preferred decision at node F (where the test says the reservoir is small) is to build
a small facility. We represent this by crossing out the inferior decisions as shown in
Figure 15.12. Doing so turns chance node D into a simple lottery with two possible
outcomes and payoffs, each with a probability of 0.50. If the test says the reservoir
is large and the firm builds a large facility, the payoff is $50 million; if the test says
the reservoir is small and the firm builds a small facility, the payoff is $20 million.
The expected payoff of this lottery is (0.5 $50 million) (0.5 $20 million)
$35 million.
Now we can simplify the tree as shown in Figure 15.13, where we have again re-
placed the payoffs for each outcome with the expected payoff for each lottery and then
folded the expected payoffs back over the lotteries. Once again, it is easy to evaluate
the decision tree: the optimal decision at node A is to conduct the seismic test, since
that decision leads to the highest expected payoff ($35 million, versus a $30 million
expected payoff for building a large facility without testing and a $25 million expected