Page 210 - Environment: The Science Behind the Stories

Population growth rate
Less than 0% (negative)
0–0.75%
0.75–1.50%
1.50–2.25%
2.25–3.00%
More than 3.00%

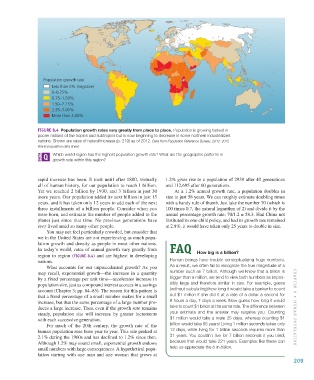
Figure 8.4 Population growth rates vary greatly from place to place. Population is growing fastest in
poorer nations of the tropics and subtropics but is now beginning to decrease in some northern industrialized
nations. Shown are rates of natural increase (p. 219) as of 2012. Data from Population Reference Bureau, 2012. 2012
World population data sheet.
Which world region has the highest population growth rate? What are the geographic patterns in
growth rate within this region?

rapid increase has been. It took until after 1800, virtually 1.2% gives rise to a population of 2939 after 40 generations
all of human history, for our population to reach 1 billion. and 112,695 after 60 generations.
Yet we reached 2 billion by 1930, and 3 billion in just 30 At a 1.2% annual growth rate, a population doubles in
more years. Our population added its next billion in just 15 size in just 58 years. We can roughly estimate doubling times
years, and it has taken only 12 years to add each of the next with a handy rule of thumb. Just take the number 70 (which is
three installments of a billion people. Consider when you 100 times 0.7, the natural logarithm of 2) and divide it by the
were born, and estimate the number of people added to the annual percentage growth rate: 70/1.2 = 58.3. Had China not
planet just since that time. No previous generations have instituted its one-child policy, and had its growth rate remained
ever lived amid so many other people. at 2.8%, it would have taken only 25 years to double in size.
You may not feel particularly crowded, but consider that
we in the United States are not experiencing as much popu-
lation growth and density as people in most other nations.
In today’s world, rates of annual growth vary greatly from FaQ How big is a billion?
region to region (Figure 8.4) and are highest in developing
nations. Human beings have trouble conceptualizing huge numbers.
What accounts for our unprecedented growth? As you As a result, we often fail to recognize the true magnitude of a
may recall, exponential growth—the increase in a quantity number such as 7 billion. Although we know that a billion is
by a fixed percentage per unit time—accelerates increase in bigger than a million, we tend to view both numbers as impos-
population size, just as compound interest accrues in a savings sibly large and therefore similar in size. For example, guess
account (Chapter 3; pp. 84–85). The reason for this pattern is (without calculating) how long it would take a banker to count
that a fixed percentage of a small number makes for a small out $1 million if she did it at a rate of a dollar a second for
increase, but that the same percentage of a large number pro- 8 hours a day, 7 days a week. Now guess how long it would
duces a large increase. Thus, even if the growth rate remains take to count $1 billion at the same rate. The difference between CHAPTER 8 • Hum A n Po P ul AT i on
steady, population size will increase by greater increments your estimate and the answer may surprise you. Counting
with each successive generation. $1 million would take a mere 35 days, whereas counting $1
For much of the 20th century, the growth rate of the billion would take 95 years! Living 1 million seconds takes only
human population rose from year to year. This rate peaked at 12 days, while living for 1 billion seconds requires more than
2.1% during the 1960s and has declined to 1.2% since then. 31 years. You couldn’t live for 7 billion seconds if you tried,
Although 1.2% may sound small, exponential growth endows because that would take 221 years. Examples like these can
small numbers with large consequences. A hypothetical popu- help us appreciate the b in billion.
lation starting with one man and one woman that grows at
209

M08_WITH7428_05_SE_C08.indd 209 12/12/14 2:58 PM