Page 195 - Fiber Optic Communications Fund

176 Fiber Optic Communications

11 00 10 01
1 1 0 0 1 0 0 1
3π
Phase (rad) π 2 π Phase (rad) π

2
t t
T s
T b
(a) (b)
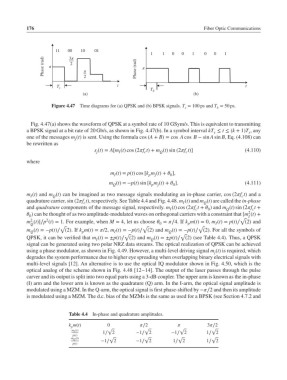
Figure 4.47 Time diagrams for (a) QPSK and (b) BPSK signals. T = 100 ps and T = 50 ps.
b
s
Fig. 4.47(a) shows the waveform of QPSK at a symbol rate of 10 GSym/s. This is equivalent to transmitting
a BPSK signal at a bit rate of 20 Gb/s, as shown in Fig. 4.47(b). In a symbol interval kT ≤ t ≤ (k + 1)T ,any
s s
one of the messages m (t) is sent. Using the formula cos (A + B)= cos A cos B − sin A sin B, Eq. (4.108) can
j
be rewritten as
s (t)= A[m (t) cos (2f t)+ m (t) sin (2f t)] (4.110)
j I c Q c
where

m (t)= p(t) cos [k m (t)+ ],
I p j 0
m (t)=−p(t) sin [k m (t)+ ]. (4.111)
Q p j 0
m (t) and m (t) can be imagined as two message signals modulating an in-phase carrier, cos (2f t) and a
I Q c
quadrature carrier, sin (2f t), respectively. See Table 4.4 and Fig. 4.48. m (t) and m (t) are called the in-phase
c I Q
and quadrature components of the message signal, respectively. m (t) cos (2f t + ) and m (t) sin (2f t +
I c 0 Q c
2
) can be thought of as two amplitude-modulated waves on orthogonal carriers with a constraint that [m (t)+
0
√ I
2
2
m (t)]∕p (t)= 1. For example, when M = 4, let us choose = ∕4. If k m(t)= 0, m (t)= p(t)∕ (2) and
Q 0 p I
√ √ √
m (t)=−p(t)∕ (2).If k m(t)= /2, m (t)=−p(t)∕ (2) and m (t)=−p(t)∕ (2). For all the symbols of
Q
I
Q
p
√ √
QPSK, it can be verified that m (t)=±p(t)∕ (2) and m (t)=±p(t)∕ (2) (see Table 4.4). Thus, a QPSK
Q
I
signal can be generated using two polar NRZ data streams. The optical realization of QPSK can be achieved
using a phase modulator, as shown in Fig. 4.49. However, a multi-level driving signal m (t) is required, which
j
degrades the system performance due to higher eye spreading when overlapping binary electrical signals with
multi-level signals [12]. An alternative is to use the optical IQ modulator shown in Fig. 4.50, which is the
optical analog of the scheme shown in Fig. 4.48 [12–14]. The output of the laser passes through the pulse
carver and its output is split into two equal parts using a 3-dB coupler. The upper arm is known as the in-phase
(I) arm and the lower arm is known as the quadrature (Q) arm. In the I-arm, the optical signal amplitude is
modulated using a MZM. In the Q-arm, the optical signal is first phase-shifted by −∕2 and then its amplitude
is modulated using a MZM. The d.c. bias of the MZMs is the same as used for a BPSK (see Section 4.7.2 and
Table 4.4 In-phase and quadrature amplitudes.
k m(t) 0 ∕2 3∕2
p
m I (t) √ √ √ √
1∕ 2 −1∕ 2 −1∕ 2 1∕ 2
p(t)
m Q (t) √ √ √ √
−1∕ 2 −1∕ 2 1∕ 2 1∕ 2
p(t)