Page 190 - Fiber Optic Communications Fund

Optical Modulators and Modulation Schemes 171

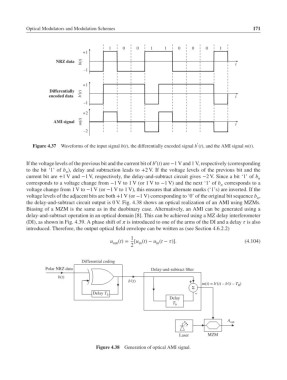
1 0 0 1 1 0 0 0 1
+1
b(t) t
NRZ data
–1

+1
Differentially
encoded data b'(t) t
–1

+2
AMI signal m(t) t
–2

′
Figure 4.37 Waveforms of the input signal b(t), the differentially encoded signal b (t), and the AMI signal m(t).

′
If the voltage levels of the previous bit and the current bit of b (t) are −1 V and 1 V, respectively (corresponding
to the bit ‘1’ of b ), delay and subtraction leads to +2 V. If the voltage levels of the previous bit and the
n
current bit are +1 V and −1 V, respectively, the delay-and-subtract circuit gives −2 V. Since a bit ‘1’ of b n
corresponds to a voltage change from −1 V to 1 V (or 1 V to −1 V) and the next ‘1’ of b corresponds to a
n
voltage change from 1 V to −1 V (or −1 V to 1 V), this ensures that alternate marks (‘1’s) are inverted. If the
voltage levels of the adjacent bits are both +1 V (or −1 V) corresponding to ‘0’ of the original bit sequence b ,
n
the delay-and-subtract circuit output is 0 V. Fig. 4.38 shows an optical realization of an AMI using MZMs.
Biasing of a MZM is the same as in the duobinary case. Alternatively, an AMI can be generated using a
delay-and-subtract operation in an optical domain [8]. This can be achieved using a MZ delay interferometer
(DI), as shown in Fig. 4.39. A phase shift of is introduced to one of the arms of the DI and a delay is also
introduced. Therefore, the output optical field envelope can be written as (see Section 4.6.2.2)
1
u (t)= [u (t)− u (t − )]. (4.104)
out in in
2

Differential coding
Polar NRZ data Delay-and-subtract filter
b(t)
b'(t) +
m(t) = b'(t) – b'(t – T B )
Σ
–
Delay T b
Delay
T b

A out
Laser MZM

Figure 4.38 Generation of optical AMI signal.