Page 28 - FINAL CFA I SLIDES JUNE 2019 DAY 3

LOS 9.n: Calculate and interpret an updated Session Unit 2:
probability using Bayes’ formula, p.191
9. Probability Concepts

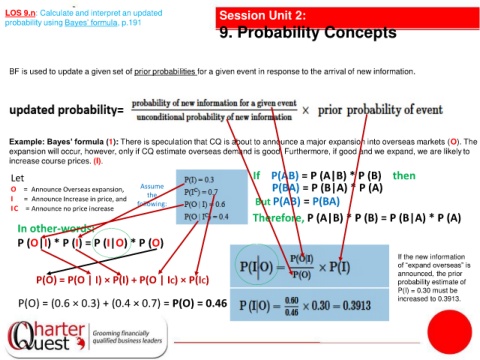
BF is used to update a given set of prior probabilities for a given event in response to the arrival of new information.

Example: Bayes’ formula (1): There is speculation that CQ is about to announce a major expansion into overseas markets (O). The
expansion will occur, however, only if CQ estimate overseas demand is good. Furthermore, if good and we expand, we are likely to
increase course prices. (I). r

Let If P(AB) = P (A|B) * P (B) then

O = Announce Overseas expansion, Assume P(BA) = P (B|A) * P (A)
I = Announce Increase in price, and the But P(AB) = P(BA)
following:
I C = Announce no price increase
Therefore, P (A|B) * P (B) = P (B|A) * P (A)
In other-words:
P (O|I) * P (I) = P (I|O) * P (O)

If the new information
of “expand overseas” is
announced, the prior
P(O) = P(O | I) × P(I) + P(O | IC) × P(IC) probability estimate of
P(I) = 0.30 must be
P(O) = (0.6 × 0.3) + (0.4 × 0.7) = P(O) = 0.46 increased to 0.3913.