Page 30 - FINAL CFA I SLIDES JUNE 2019 DAY 3

LOS 9.o: Identify the most appropriate method to Session Unit 2:
solve a particular counting problem and solve
counting problems using factorial, combination, and 9. Probability Concepts
permutation concepts, p.193

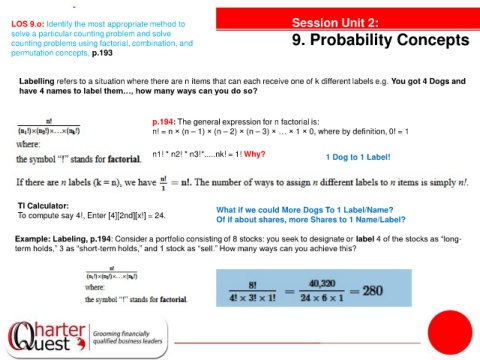
Labelling refers to a situation where there are n items that can each receive one of k different labels e.g. You got 4 Dogs and
have 4 names to label them…, how many ways can you do so?

p.194: The general expression for n factorial is:
n! = n × (n – 1) × (n – 2) × (n – 3) × … × 1 × 0, where by definition, 0! = 1

n1! * n2! * n3!*.....nk! = 1! Why? 1 Dog to 1 Label!

TI Calculator: What if we could More Dogs To 1 Label/Name?
To compute say 4!, Enter [4][2nd][x!] = 24.
Of if about shares, more Shares to 1 Name/Label?

Example: Labeling, p.194: Consider a portfolio consisting of 8 stocks: you seek to designate or label 4 of the stocks as “long-
term holds,” 3 as “short-term holds,” and 1 stock as “sell.” How many ways can you achieve this?