Page 199 - Maxwell House

SOLUTION OF BASIC EQUATIONS OF ELECTRODYNAMICS 179

4.2 RADIATION OF ELECTROMAGNETIC WAVES

4.2.1 Introduction

First we have to choose between the space-time and space-frequency domain analysis. In
general, the space-frequency approach is more transparent and leads to slightly modest
equations. Clearly, we can come back to the space-time domain solution using Fourier
transform like (1.88) from Chapter 1. So the space-time domain and space-frequency domain
are just two equivalent ways of looking at the same electromagnetic process.
4.2.2 Radiation EM Waves by Infinitesimal Current Element

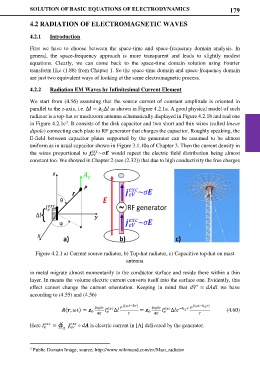
We start from (4.56) assuming that the source current of constant amplitude is oriented in
parallel to the z-axis, i.e. ∆ = ∆ as shown in Figure 4.2.1a. A good physical model of such
0
radiator is a top-hat or mushroom antenna schematically displayed in Figure 4.2.1b and real one
3
in Figure 4.2.1c . It consists of the disk capacitor and two short and thin wires (called linear
dipole) connecting each plate to RF generator that charges the capacitor. Roughly speaking, the
E-field between capacitor plates supported by the generator can be assumed to be almost
uniform as in usual capacitor shown in Figure 3.1.10a of Chapter 3. Then the current density in
the wires proportional to ~ would repeat the electric field distribution being almost

constant too. We showed in Chapter 2 (see (2.32)) that due to high conductivity the free charges

Figure 4.2.1 a) Current source radiator, b) Top-hat radiator, c) Capacitive top-hat on mast
antenna
in metal migrate almost momentarily to the conductor surface and reside there within a thin
layer. In means the volume electric current converts itself into the surface one. Evidently, this
effect cannot change the current orientation. Keeping in mind that = we have
′
according to (4.55) and (4.56)

�− � � (− 1 )
0 0 − 2
(, ) = 0 ∆ = 0 ∆ (4.60)
4 4
Here = ∯ ∘ is electric current in [A] delivered by the generator.

3 Public Domain Image, source: http://www.wikiwand.com/en/Mast_radiator