Page 202 - Maxwell House

182 Chapter 4

0 ′ �− � � �− � �
′
′
(, ) ≅ � ∫ ( ) � 0 ∘ � = (, , ) (4.66)
4 ′
Here (, ) is some independent of time and coordinate vector potential. It is clear that in
the far-field zone the expanding wavefront of waves emitted by any kind of antennas is
spherical. It is worthwhile to point out that the shape of big radius sphere can be approximated
very closely by a polygon (green surface sells) as it can be seen in Figure 4.2.4a. If so, the
wavefront within each such relatively small part can be treated as a plane. The wave with the
uniform phase distribution over a limited plane is called the plane wave. According to (4.66)
true plane waves of infinite extend never exist in nature.

4.3 ELEMENTARY RADIATORS

4.3.1 Electric and Magnetic Fields Emitted by Infinitesimal Current Element

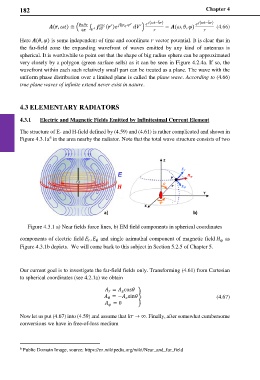
The structure of E- and H-field defined by (4.59) and (4.61) is rather complicated and shown in
6
Figure 4.3.1a in the area nearby the radiator. Note that the total wave structure consists of two

~

a) b)

Figure 4.3.1 a) Near fields force lines, b) EM field components in spherical coordinates

components of electric field , and single azimuthal component of magnetic field as

Figure 4.3.1b depicts. We will come back to this subject in Section 5.2.5 of Chapter 5.

Our current goal is to investigate the far-field fields only. Transforming (4.61) from Cartesian
to spherical coordinates (see 4.2.1a) we obtain

= cos

= − sin� (4.67)

= 0

Now let us put (4.67) into (4.59) and assume that → ∞. Finally, after somewhat cumbersome
conversions we have in free-of-loss medium

6 Public Domain Image, source: https://en.wikipedia.org/wiki/Near_and_far_field