Page 203 - Maxwell House

SOLUTION OF BASIC EQUATIONS OF ELECTRODYNAMICS 183

∆ (−)
= sin ⎫
0

2 ⎪
(4.68)
∆ (−)
= sin

2 ⎬
⎪
= = = = 0 ⎭

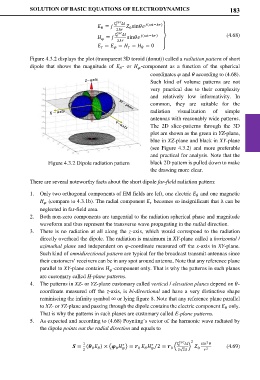
Figure 4.3.2 displays the plot (transparent 3D toroid (donut)) called a radiation pattern of short
dipole that shows the magnitude of - or -component as a function of the spherical

coordinates and according to (4.68).
Such kind of volume patterns are not
very practical due to their complexity
and relatively low informativity. In
common, they are suitable for the
radiation visualization of simple
antennas with reasonably wide patterns.
The 2D slice-patterns through the 3D
plot are shown as the green in YZ-plane,
blue in XZ-plane and black in XY-plane
(see Figure 4.3.2) and more preferable
and practical for analysis. Note that the
Figure 4.3.2 Dipole radiation pattern black 2D pattern is pulled down to make
the drawing more clear.
There are several noteworthy facts about the short dipole far-field radiation pattern:

1. Only two orthogonal components of EM fields are left, one electric and one magnetic

(compare to 4.3.1b). The radial component becomes so insignificant that it can be

neglected in far-field area.
2. Both non-zero components are tangential to the radiation spherical phase and magnitude
waveform and thus represent the transverse wave propagating in the radial direction.
3. There is no radiation at all along the z-axis, which would correspond to the radiation
directly overhead the dipole. The radiation is maximum in XY-plane called a horizontal /
azimuthal plane and independent on -coordinate measured off the x-axis in XY-plane.
Such kind of omnidirectional pattern are typical for the broadcast transmit antennas since
their customers’ receivers can be in any spot around antenna. Note that any reference plane
parallel to XY-plane contains -component only. That is why the patterns in such planes

are customary called H-plane patterns.
4. The patterns in XZ- or YZ-plane customary called vertical / elevation planes depend on -
coordinate measured off the z-axis, is bi-directional and have a very distinctive shape
reminiscing the infinity symbol ∞ or lying figure 8. Note that any reference plane parallel
to XZ- or YZ-plane and passing through the dipole contains the electric component only.

That is why the patterns in such planes are customary called E-plane patterns.
5. As expected and according to (4.68) Poynting’s vector of the harmonic wave radiated by
the dipole points out the radial direction and equals to

2 2
1 ∆ sin
∗
∗
= ( ) × � � = ⁄ = � � (4.69)
2
2 0 0 0 0 2√2 0 2